size weight illusion experiment

An official website of the United States government

Official websites use .gov A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS A lock ( Lock Locked padlock icon ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

• Publications
• Account settings
• Advanced Search
• Journal List

Mass Is All That Matters in the Size–Weight Illusion

Myrthe a plaisier, jeroen b j smeets.

• Author information
• Article notes
• Copyright and License information

* E-mail: [email protected]

Competing Interests: The authors have declared that no competing interests exist.

Conceived and designed the experiments: MAP JBJS. Performed the experiments: MAP. Analyzed the data: MAP. Wrote the paper: MAP.

Received 2011 Nov 9; Accepted 2012 Jul 10; Collection date 2012.

This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are properly credited.

An object in outer space is weightless due to the absence of gravity, but astronauts can still judge whether one object is heavier than another one by accelerating the object. How heavy an object feels depends on the exploration mode: an object is perceived as heavier when holding it against the pull of gravity than when accelerating it. At the same time, perceiving an object’s size influences the percept: small objects feel heavier than large objects with the same mass (size–weight illusion). Does this effect depend on perception of the pull of gravity? To answer this question, objects were suspended from a long wire and participants were asked to push an object and rate its heaviness. This way the contribution of gravitational forces on the percept was minimised. Our results show that weight is not at all necessary for the illusion because the size–weight illusion occurred without perception of weight. The magnitude of the illusion was independent of whether inertial or gravitational forces were perceived. We conclude that the size–weight illusion does not depend on prior knowledge about weights of object, but instead on a more general knowledge about the mass of objects, independent of the contribution of gravity. Consequently, the size–weight illusion will have the same magnitude on Earth as it should have on the Moon or even under conditions of weightlessness.

Introduction

The size-weight illusion is the well-known effect that large objects are perceived to be lighter than small objects of the same weight [1] . Although this illusion originally was discovered as a multi–sensory phenomenon in which the visually perceived size of an object influences its perceived weight, the illusion also occurs in the absence of vision if haptic size cues are available [2] . This shows that the illusion is not a multi-sensory one per se. It is based on a general effect of perceived size on perceived weight. In fact, showing an object before lifting, but not during lifting already triggers the illusion [3] . Similarly, there exists also a material–weight illusion [4] , [5] : objects that are perceived to be made out of a denser material are perceived to be lighter than objects with the same size and weight that appear to be made out of a less dense material. Apparently, our percept of how heavy an object feels is biased by prior knowledge about the general relationship between object properties and the weight of an object. This suggests that the size–weight illusion occurs because we have learned that there is a correlation between size and weight. This idea is supported by a study in which it was shown that the illusion can be reversed: repeatedly lifting a set of objects manufactured such that the smaller objects had more mass than the larger objects for several days reduced the illusion and finally reversed it [6] .

Combining information sources, such as size and weight, is common in human perception. It has been shown that when judging the size of an object through vision and touch simultaneously, the two estimates are integrated in a statistically optimal fashion [7] . This means that the combined percept is more precise than either of the two percepts independently. In fact, one can even learn to integrate two unrelated perceptual signals such as stiffness and luminance [8] . Sometimes, several information sources are combined with prior assumptions. This can be modelled using Bayesian statistics [9] . In the case of the size–weight illusion a perceptual estimate of size is combined with an estimate of the weight together with a prior for large objects being heavier. The way these information sources are combined in the size–weight illusion is fundamentally different from the previous examples, as it makes the percept less accurate and can be regarded as anti-Bayesian [10] .

A prior for larger objects being heavier would suggest that a larger lifting force is applied for lifting large objects than for lifting small objects [11] . It has been shown that initially larger lifting forces are applied when lifting large objects, but the difference in the applied forces disappears within a few lifts while the perceived weight difference remains constant [12] , [13] . This suggests that the illusion is not caused by applying more force when lifting a large object than when lifting a smaller object.

Since the illusion is usually referred to as size-weight illusion, one would expect it to be related to the weight of an object. Note that weight is another word for the gravitational force acting upon an object, which is proportional to the (gravitational) mass of an object. The mass of an object can also be experienced without weight through inertial forces proportional to the (inertial) mass acting during acceleration of an object [14] . This is why the mass of an object can be judged in the absence of gravity, such as in outer–space. Since gravitational and inertial masses of an object are the same (Einstein’s equivalence principle), one might expect that the two types of mass appear to be the same for the perceptual system. Surprisingly, an object is perceived to be almost twice as heavy through perception of gravitational pull than through perception of inertial forces [15] , [16] . So, clearly the perceived heaviness of an object depends on whether inertial or gravitational forces are perceived, even though the underlying object property, mass, is the same.

In the present study we investigated whether the size-weight illusion depends on perceiving the pull of gravity, i.e. whether it is caused by a prior for weight or a by more general prior for the mass of an object. To this end we investigated whether the size–weight illusion occurs in the absence of weight through perception of inertial forces only. If the size–weight illusion occurs independent of gravitational forces, the illusion must be related to the mass of an object independent of the forces acting upon it. One reason for not expecting the illusion to occur in the absence of gravitational forces is that in daily life we rarely experience the heaviness of an object without perceiving gravitational forces. This means that the prior for larger objects being heavier may be limited to perception of gravitational forces, i.e. weight. Secondly, for perceiving inertial mass it is necessary to combine information about the acceleration of an object with efferent or afferent information about the applied amount of force. Gravitational forces (weight), in contrast, can be perceived purely tactual through the pressure of an object on the skin of the static hand. Therefore, fundamentally different sources of information are being used for perception of mass through inertial or gravitational forces.

To investigate whether the size–weight illusion occurs in the absence of weight, a set of objects differing in size but with the same mass was constructed. To let participants perceive inertial forces only, we suspended the objects from a long pair of wires and asked the participant to give the objects a short push after which they rated the perceived heaviness. This way perception of gravitational forces acting on the object was minimised. We let participants perform this task with and without visual feedback of the trajectory of the object after pushing to test whether participants used visual information about how far the object travelled. Finally, we also asked participants to rate heaviness after lifting the objects and placing them back as a control task. This allowed us to test whether the magnitude of the illusion as obtained through perception of inertial forces differed from the illusion obtained in the traditional way.

Materials and Methods

Participants.

Twenty self-reported right-handed participants volunteered in the experiment (age range 22 to 40 years). All participants were naive as to the purpose of the experiment. Half of the participants performed the experiment pushing the objects with full vision. The other half also pushed the objects, but without visual feedback of the object’s trajectory. Eight of the subjects that performed the pushing with full visual feedback task also performed the control task of lifting with full vision.

Ethics Statement

The experiment was conducted as part of a program that was approved by the ethical committee of the Faculty of Human Movement Sciences of VU University. All participants signed a statement of informed consent prior to participation in the experiments.

The stimuli consisted of a set of four objects constructed out of MDF (medium density fibre). Three test objects differing in size (small, medium, large) were weighed down such that the mass of each object was 250 g ( Fig. 1A ). The fourth object (reference) had the same dimensions as the medium sized test object, but had a mass of 200 g. The ratings for the two medium sized objects were used to convert the heaviness ratings of the other objects into grams.

Figure 1. Description of the stimuli and set-up.

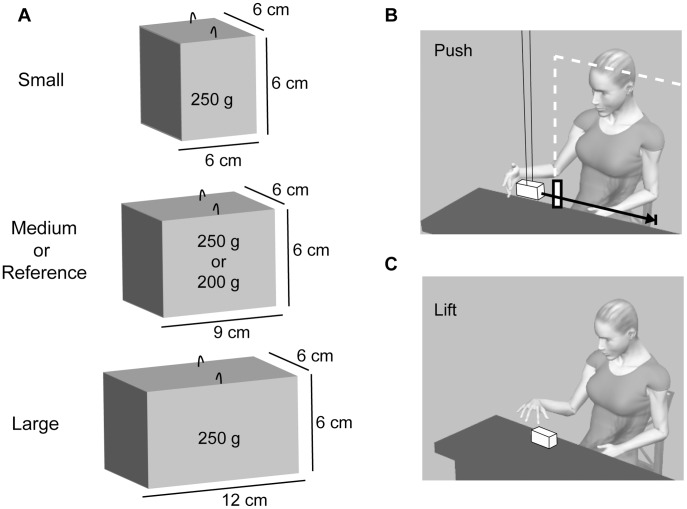
(A) Dimensions of the four objects constructed out of medium density fibre (MDF). The three test objects (small, medium, large) had a mass of 250 g, the reference object had a mass of 200 g. Each object had two hooks attached to the top surface such that they could be suspended from a double wire attached to the ceiling. (B) A schematic representation of the set up. Subjects were seated in front of a table above which the objects could be suspended one at a time. The distance from the object to the ceiling (the effective length of the pendulum) was 2.3 m. The subjects were asked to push the object such that it travelled over an indicated distance of 50 cm (black arrow). Either an obstacle (withe bar; group with visual feedback) or a screen (white outline; group without visual feedback) ensured that the participant could not keep contact with the object for a distance larger than 10 cm. The experimenter caught and replaced the object after each push. (C) In the lifting task an object was placed on the table in front of the participant and he or she was asked to lift the object to a marked height (20 cm) and place it back on the table. The objects were always grasped on the horizontal 6 cm axes, such that grip aperture was the same for all object sizes.

A small infrared Light Emitting Diode was attached in the centre of each object’s surface facing away from the subject. The position of the object was recorded at 500 Hz using an Optotrak position tracking system (Northern Digital). These data were used to calculate the objects’ velocities.

In the pushing task the objects were suspended just above table height from a pair of long wires in front of the participant. The participants were asked to give the object a short push such that it travelled over a distance of 50 cm ( Fig. 1B ) and rate how heavy the object felt using arbitrary numbers (i.e. method of absolute magnitude estimation [17] ). These ratings were converted into z-scores by taking the difference between the individual ratings of a participant and his or her average rating, before dividing by the standard deviation of the ratings. For the task without visual feedback a screen was placed in front of the subject, such that the object was initially visual, but it disappeared behind the screen shortly after the push.

The procedure in the control task was the same as in the pushing task, but now participants were instructed to lift the objects between their thumb and index finger. They lifted the objects grasping them in the centre along the 6 cm axis, such that grip aperture was the same for all objects ( Fig. 1C ).

In all tasks the test objects were presented in 15 sets of three trials; in every set each object was presented once and the order of presentation within each set was randomised. After these 15 sets of three trials, another 10 trials were performed in which the reference object was presented 5 times randomly interleaved with 5 times one of the test objects. All trials were performed in one continuous run such that subjects were not aware of the introduction of the reference object.

Repeated measures ANOVA was performed on the z -scores of the heaviness ratings with object size as a repeated factor and visual feedback as a between subjects factor. The effect of object size was also tested with a repeated measures ANOVA on the peak velocities of the objects. Finally, a repeated measures ANOVA with object size and task as repeated factors was performed to compare the lifting and pushing with visual feedback conditions.

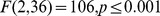
Figure 2. Heaviness ratings averaged over subjects.

(A) Judged mass for pushing the objects with full visual feedback expressed as z-scores. (B) Judged mass expressed in grams for pushing with full visual feedback (black) and pushing without visual feedback of the trajectory (dark grey). The scores were normalised in such a way that the judgments for the medium and reference object corresponded to 250 g and 200 g, respectively, so that the standard error for these objects is zero. (C) Judged mass expressed in grams for subjects that performed both the pushing with full visual feedback (black) as well as the lifting (grey) task. In all panels the error bars represent the between–subjects standard error.

Figure 3. Heaviness ratings and peak velocities of the objects.

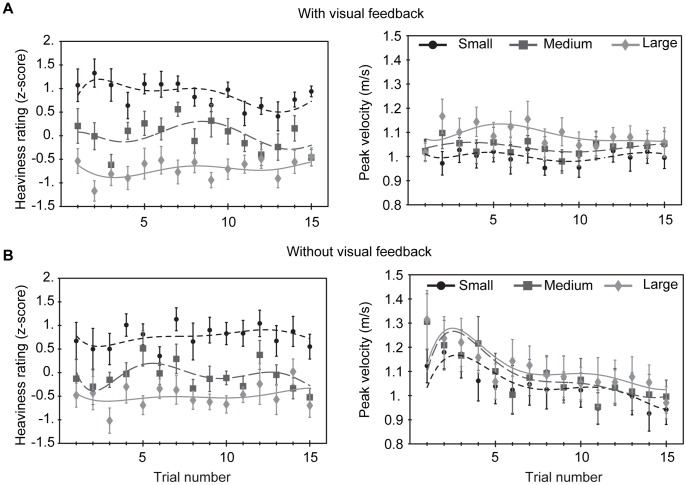
Ratings and velocities are shown for each trial with (A) and without (B) visual feedback of the objects’ trajectories. The lines represent polynomials that were fitted to the data solely as a guide for the eye.

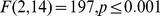
First, we have shown that perception of weight is not at all necessary for the size–weight illusion to occur. The illusion should therefore be interpreted as a size–mass illusion. The results also show that the magnitude of the illusion was similar for lifting and pushing. This demonstrates that weight is not only unnecessary for the illusion to occur, but that also the magnitude of the illusion does not depend on providing weight as a cue. An explanation for the fact that the illusion size reported here is large compared to what has been reported previously is that we have used a set containing one cube, while the other objects had the same height and depth but were elongated compared to the cube. In other studies usually a set of cubes in different sizes was used. Possibly the visual estimate of the volume differences between the shapes was therefore more pronounced in our study. Anyway, the constancy of the magnitude of the illusion across lifting and pushing indicates that the size–mass illusion is independent of the basis of the heaviness percept.

Our results show that the objects with different sizes reached different peak velocities. There was no adaptation of the peak velocities over trials, i.e. participants didn’t adapt their pushing force such that all object sizes were pushed with the same amount of force after a number of trials. It has been shown that the maximum grip and load force rates adapt within as little as five trials such that there are no differences in these values anymore for lifting small and large objects [12] . This suggests that a mismatch between lifting force and an object’s weight is not an explanation for the size–weight illusion. It has, however, also been shown that the grip and load forces themselves do not adapt or at least do not adapt as fast as the peak grip and load force rates [18] . Since the peak velocity of the objects in our study is directly coupled to the amount of force applied (and not to its rate), adaptation is not to be expected for the peak velocities.

Generally, the size-weight illusion is explained in terms of a discrepancy between prior expectations and sensory information [6] , [10] . This means that the perceptual system uses knowledge from prior experiences such as that larger objects are heavier than smaller ones [6] . Therefore different forces are used to lift small and large objects with the same mass [11] , [12] . In daily life situations we perceive either only gravitational forces when statically holding an object or a combination of gravitational and inertial forces while moving an object, but we rarely experience inertial forces alone. Nonetheless, we found that prior experience handling objects results in the same illusion magnitude for lifting and pushing of objects. This shows that the size–weight illusion is not caused by a perceptual prior for the lifting forces directly associated with weight, but by a more general prior related to the mass of objects instead.

Several researchers have used the size–weight illusion to investigate the mechanisms underlying heaviness perception [19] , [20] . The present study demonstrates that the size–weight illusion is very robust, and is independent of the mechanism underlying the heaviness percept. We found that the magnitude of the illusion is the same for lifting and pushing whereas the heaviness percept (and the mechanism it is based on) differs between these modes of exploration. Apparently, there is a discrepancy, because both modes of exploration show a large difference in perceived heaviness, but not in the magnitude of the size–weight illusion. This indicates that one should be careful when drawing conclusion about heaviness perception in general from results obtained from studying size–weight illusion.

In the physical world gravitational and inertial mass are the same according to Einstein’s equivalence principle. Mass is, however, perceived differently through inertial forces than through gravitational forces [15] . An explanation is that the perceptual system treats gravitational and inertial mass differently and thereby violates the equivalence principle [16] . Here we have shown that this violation is limited as the size–weight illusion is based on a prior for mass, regardless whether mass is perceived through gravitation or inertia. Therefore, we conclude that the illusion is based on a prior for the mass of an object, not a prior for forces acting on the object.

Funding Statement

Funding provided by VICI grant (MaGW 453-08-004) for JBJS from the Netherlands Organization for Scientific Research. Rubicon grant from NWO in collaboration with the Marie Curie Cofund (MAGW 446-10-012) for MAP. www.nwo.nl . The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.

• 1. Charpentier A (1891) Analyse experimentale: De quelques elements de la sensation de poids. Archives de Physiologie Normale et Pathologique 3: 122–135. [ Google Scholar ]
• 2. Ellis RR, Lederman SJ (1993) The role of haptic versus visual volume cues in the size weight illusion. Perception & Psychophysics 53: 315–324. [ DOI ] [ PubMed ] [ Google Scholar ]
• 3. Buckingham G, Goodale MA (2010) Lifting without Seeing: The Role of Vision in Perceiving and Acting upon the Size Weight Illusion. PLOS ONE 5 e9709. [ DOI ] [ PMC free article ] [ PubMed ] [ Google Scholar ]
• 4. Ellis R, Lederman S (1999) The material-weight illusion revisited. Perception & Psychophysics 61: 1564–1576. [ DOI ] [ PubMed ] [ Google Scholar ]
• 5. Buckingham G, Cant JS, Goodale MA (2009) Living in A Material World: How Visual Cues to Material Properties Affect the Way That We Lift Objects and Perceive Their Weight. Journal of Neurophysiology 102: 3111–3118. [ DOI ] [ PubMed ] [ Google Scholar ]
• 6. Flanagan JR, Bittner JP, Johansson RS (2008) Experience Can Change Distinct Size-Weight Priors Engaged in Lifting Objects and Judging their Weights. Current Biology 8: 1742–1747. [ DOI ] [ PubMed ] [ Google Scholar ]
• 7. Ernst MO, Banks MS (2002) Humans integrate visual and haptic information in a statistically optimal fashion. Nature 415: 429–433. [ DOI ] [ PubMed ] [ Google Scholar ]
• 8. Ernst MO (2007) Learning to integrate arbitrary signals from vision and touch. Journal of Vision 7. [ DOI ] [ PubMed ]
• 9. Weiss Y, Simoncelli E, Adelson E (2002) Motion illusions as optimal percepts. Nature Neuroscience 5: 598–604. [ DOI ] [ PubMed ] [ Google Scholar ]
• 10. Brayanov JB, Smith MA (2010) Bayesian and “Anti-Bayesian” Biases in Sensory Integration for Action and Perception in the Size-Weight Illusion. Journal of Neurophysiology 103: 1518–1531. [ DOI ] [ PMC free article ] [ PubMed ] [ Google Scholar ]
• 11. Gordon AM, Forssberg H, Johansson RS, Westling G (1991) Visual force cues in the programming of manipulative forces during precision grip. Experimental Brain Research 83: 477–482. [ DOI ] [ PubMed ] [ Google Scholar ]
• 12. Flanagan JR, Beltzner MA (2000) Independence of perceptual and sensorimotor predictions in the size-weight illusion. Nature Neuroscience 3: 737–741. [ DOI ] [ PubMed ] [ Google Scholar ]
• 13. Grandy M, Westwood D (2006) Opposite perceptual and sensorimotor responses to a size-weight illusion. Journal of Neurophysiology 95: 3887–3892. [ DOI ] [ PubMed ] [ Google Scholar ]
• 14. Ross HE, Brodie EE, Benson AJ (1984) Mass discrimination during prolonged weightlessness. Science 225: 219–221. [ DOI ] [ PubMed ] [ Google Scholar ]
• 15. Ross HE, Brodie EE (1987) Weber fractions for weight and mass as a function of stimulus-intensity. Quarterly Journal of Experimental Psychology 39: 77–88. [ DOI ] [ PubMed ] [ Google Scholar ]
• 16. Bergmann Tiest WM, Kappers AML (2010) Haptic perception of gravitational and inertial mass. Attention, Perception & Psychophysics 72: 1144–1154. [ DOI ] [ PubMed ] [ Google Scholar ]
• 17. Zwislocki JJ, Goodman DA (1980) Absolute scaling of sensory magnitudes - a validation. Perception & Psychophysics 28: 28–38. [ DOI ] [ PubMed ] [ Google Scholar ]
• 18. Buckingham G, Goodale MA (2010) The influence of competing perceptual and motor priors in the context of the size-weight illusion. Experimental Brain Research 205: 283–288. [ DOI ] [ PubMed ] [ Google Scholar ]
• 19. Amazeen E, Turvey M (1996) Weight perception and the haptic size weight illusion are functions of the inertia tensor. Journal of Experimental Psychology: Human Perception and Performance 22: 213–232. [ DOI ] [ PubMed ] [ Google Scholar ]
• 20. Zhu Q, Bingham GP (2011) Human readiness to throw: the size-weight illusion is not an illusion when picking the best objects to throw. Evolution and Human Behavior 32: 288–293. [ Google Scholar ]
• View on publisher site
• PDF (509.3 KB)
• Collections

Similar articles

Cited by other articles, links to ncbi databases.

• Download .nbib .nbib
• Format: AMA APA MLA NLM

Add to Collections

A meta-analysis of the size-weight and material-weight illusions

• Theoretical Review
• Published: 01 May 2019
• Volume 26 , pages 1195–1212, ( 2019 )

Cite this article

• Elizabeth J. Saccone   ORCID: orcid.org/0000-0001-5763-6198 1 ,
• Oriane Landry 1 &
• Philippe A. Chouinard 1  

4074 Accesses

16 Citations

11 Altmetric

Explore all metrics

The current study comprises the first systematic meta-analysis of weight illusions. We obtained descriptive data from studies in which subjective heaviness estimates were made for pairs or groups of objects that had the same mass and different volumes (size–weight illusion; SWI) or different apparent material properties (material–weight illusion; MWI). Using these data, we calculated mean effect sizes to represent illusion strength. Other study details, including stimulus mass, volume, density, and degree of visual and somatosensory access to the stimuli were also recorded to quantify the contribution of these variables to effect sizes for the SWI. The results indicate that the SWI has a larger mean effect size than the MWI and that the former is consistent in strength when information about stimulus size is gained through somatosensory channels, regardless of visual access. The SWI is weaker when only the visual system provides size information. Effect sizes for the SWI were larger when there was a greater difference in volume across the stimuli. There was also a positive correlation between SWI strength and the difference in physical density across the different experimental stimuli, even after controlling for volume differences. Together, we argue that these findings provide support for theories of weight illusions that are based on conceptual expectancies as well as those that are based on bottom-up processing of physical density. We further propose that these processes, which have been considered dichotomously in the past, may not be mutually exclusive from each other and could both contribute to our perception of weight when we handle objects in everyday life.

Similar content being viewed by others

The influence of size in weight illusions is unique relative to other object features

Contribution of surface material and size to the expected versus the perceived weight of objects.

Object size can influence perceived weight independent of visual estimates of the volume of material

Avoid common mistakes on your manuscript.

Introduction

Research into weight illusions, where objects with the same mass feel different in weight, paints a complex picture of weight perception. Weight illusions demonstrate how our conscious experience of an object’s weight is subject to influence by its other features. For example, size (Charpentier, 1891 ), material composition (Seashore, 1899 ; Wolfe, 1898 ), distribution of mass (Amazeen & Turvey, 1996 ), shape (Dresslar, 1894 ; Kahrimanovic, Bergmann Tiest, & Kappers, 2011 ), and colour (De Camp, 1917 ; Walker, Francis, & Walker, 2010 ) are known to influence an object’s perceived weight. This line of research points to a complex process by which the brain considers multiple types of visual and somatosensory information to make sense of an object’s weight.

The size–weight illusion (SWI) is the most studied weight illusion. Charpentier ( 1886 , 1891 ) was the first to document the SWI, in which the smaller of two objects of equal mass is typically reported as heavier. Greater differences in size between SWI objects tend to result in greater differences in perceived weight (Ellis & Lederman, 1993 ; J. Ross & Di Lollo, 1970 ). The illusion is so robust that it is still experienced even when the perceiver is told that the two objects have the same mass (Flanagan & Beltzner, 2000 ). Likewise, the perceptual experience of the illusion persists even following motor adaptation to the objects’ true weight (Buckingham, Ranger, & Goodale, 2011b ; Chang, Flanagan, & Goodale, 2008 ; Chouinard, Large, Chang, & Goodale, 2009 ; Flanagan & Beltzner, 2000 ; Flanagan, Bittner, & Johansson, 2008 ; Grandy & Westwood, 2006 ). To explain, fingertip forces measured while participants lift two different-sized objects of the same mass typically reflect a greater degree of force applied for the larger one than for the smaller one, but only during initial lifts. Over subsequent lifts, the applied forces for the two objects become more similar as the motor system learns the objects’ real weight. Yet, the perceptual experience of the SWI endures.

There has been extensive research into weight illusions since they were first documented more a century ago. Many theories have been proposed to explain them (for reviews, see Buckingham, 2014 ; Saccone & Chouinard, 2019 ; Dijker, 2014 ); however, none can account for all relevant findings. Accordingly, the mechanisms underlying weight illusions, as well as weight perception more generally, remain unclear. Many theories of weight illusions can be classified under the broad category of conceptual expectancies, as elaborated below and in Fig. 1 . However, there is evidence that the SWI is not entirely explained by these accounts. Other accounts of weight perception propose an influence of bottom-up processing of object features (see Fig. 1 ). These processes might also contribute to the SWI. The present investigation comprises a systematic meta-analysis that aimed to shed light on these two processes, which have, at times, been treated dichotomously in previous research.

Graphical depiction of the two types of accounts that might influence weight perception in the SWI

Conceptual expectancies

Conceptual expectancy accounts of weight illusions typically emphasise how our understanding and knowledge of objects affects how we perceive their weight (see Fig. 1 ). According to these accounts, the SWI reflects our learned understanding of the relationship between size and weight (Buckingham & Goodale, 2010 , 2013 ; Buckingham & MacDonald, 2016 ; Plaisier & Smeets, 2015 ). Size is often a reliable indicator of object weight, and experience has taught us to expect that larger objects are typically heavier than smaller ones. This size–weight relationship, which frequently holds in the real world, is violated in the SWI. In this instance, a person’s sensory input during lifting contradicts their expectations based on their understanding of the relationship between size and weight. It is this contradiction that causes the smaller object to feel heavier, because it weighs more than expected, and the larger object to feel lighter, because it weighs less than expected. Some believe that expectations may contribute to perception in a top-down manner (Buckingham, 2014 ). The logic here is that some conceptual understanding of the object is computed before experiencing its weight, which then influences weight perception.

Conceptual expectancy accounts are supported by studies that manipulate expectations of object weight (Buckingham & Goodale, 2010 ; Ellis & Lederman, 1998 ; Flanagan et al., 2008 ). For example, Buckingham and Goodale ( 2010 ) produced an SWI based entirely on the expected rather than the actual size of objects. Participants were first shown a small or large cube. Then, participants’ vision was obscured, and the experimenter replaced the small or large object with a medium-sized cube, which participants lifted with a handle. Despite lifting the medium cube on every trial, the participants reported the cube as being heavier when they had previewed the smaller cube and lighter when they had previewed the larger cube, in line with the SWI. Flanagan et al. ( 2008 ) also demonstrated the influence of expectations on weight perception by contextually changing the participants’ understanding of the size–weight relationship. Participants practiced lifting objects that were large and light, or small and heavy. After extensive training with these unusual stimuli, participants were then tested on the standard SWI, using two different-sized objects with the same mass. They experienced a weak but significant reversal of the SWI, reporting that the larger object felt heavier.

Aside from the SWI, research from other weight illusions also suggests that weight perception can be driven by conceptual expectancies. One such example was provided by Ellis and Lederman ( 1998 ). They asked experienced golfers and nongolfers to rate the heaviness of real and practice golf balls that had the same mass. Importantly, only the experienced golfers knew that practice golf balls are lighter than real ones. The nongolfers, who did not expect weight variations across the different types of golf balls, did not perceive any weight differences between the real and practice balls. Conversely, the experienced golfers reported the practice balls being as heavier. This finding is consistent with the SWI, whereby it reflects the opposite perceptual experience to that predicted by the typical, expected feature–weight relationship.

The material–weight illusion (MWI; Buckingham, Cant, & Goodale, 2009 ; Baugh, Kao, Johansson, & Flanagan, 2012 ; Ellis & Lederman, 1999 ; Seashore, 1899 ; Wolfe, 1898 ) is frequently cited to support conceptual expectancy accounts of weight illusions (Buckingham, 2014 ; Buckingham et al., 2009 ; Buckingham, Ranger, & Goodale, 2011a ; Ellis & Lederman, 1999 ; H. E. Ross, 1969 ; Vicovaro & Burigana, 2017 ). The MWI is another well-studied weight illusion. In the MWI, a person lifts two objects of the same size and mass that appear to be made of different materials, such as polystyrene and aluminium (Buckingham & Goodale, 2013 ). In this context, it is the apparent material composition, rather than size, that informs weight expectations. If there are two equally sized objects made from either polystyrene or aluminium, our conceptual understanding of these materials tells us that the aluminium object should be heavier. The typical finding in the MWI is that the object apparently made from a lighter material (e.g., polystyrene) is perceived as being heavier than the other (e.g., aluminium; Baugh et al., 2012 ; Buckingham et al., 2009 ; Ellis & Lederman, 1999 ). Therefore, established material–weight associations are violated, producing the opposite perceptual experience, consistent with other weight illusions.

Overall, a conceptual expectancy account fits well with some of the weight illusion literature. However, some findings that are specific to the SWI create problems for this account, as we will discuss below. These findings suggest that a different mechanism might also contribute to the SWI.

Conceptual expectancies may not entirely explain the SWI

The first consideration is that the SWI might be stronger relative to other weight illusions (Buckingham, Bieńkiewicz, Rohrbach, & Hermsdörfer, 2015a ; Buckingham & Goodale, 2013 ; Vicovaro & Burigana, 2017 ), potentially creating a dilemma for conceptual accounts of weight illusions. According to these accounts, it is our expectations, formulated by our conceptual knowledge that drives the misperception of weight in a top-down manner (Buckingham, 2014 ; Saccone & Chouinard, 2019 ). If weight perception truly operated in a top-down manner, then the type of concept driving these expectations should not matter, provided they afford similar predictive values. In this context, does one concept exert a stronger change in perceived weight than the other? If so, is this related to differences in predictive values, as one might expect from a conceptual expectancy account, or by some other explanation?

Findings from two studies by Buckingham and colleagues indicate that the SWI is a stronger illusion than the MWI (Buckingham, Bieńkiewicz, et al., 2015; Buckingham & Goodale, 2013 ), shedding some light on this issue. In one study, Buckingham, Bieńkiewicz, et al. (2015) conducted a neuropsychological investigation of weight illusions that also included a healthy control group. Participants lifted both SWI and MWI object pairs and stated which one of each pair was heavier. An SWI was evident when the smaller object was reported as being heavier than the larger object, and the MWI was present when the polystyrene object was reported as being heavier than the aluminium one. The control group experienced an SWI in 95.8% of trials, but the MWI in only 35.1% trials. Buckingham and Goodale’s ( 2013 ) study also included both SWI and MWI stimuli. They compared the difference in heaviness ratings for the SWI (large vs small) and the MWI (polystyrene vs aluminium). The SWI appeared to be considerably stronger than the MWI, in that there was a greater difference in perceived weight between the large and small stimuli than between the volume-matched polystyrene and aluminium objects. These findings demonstrate that variations in stimulus surface material lead to smaller differences in perceived weight (Buckingham & Goodale, 2013 ) and differences that are less reliable (Buckingham, Bieńkiewicz, et al., 2015) than variations in size.

The question then arises as to whether these differences exist because size is a stronger predictor of an object’s weight. To test this possibility, Vicovaro and Burigana ( 2017 ) had participants rate both the expected weight of objects that varied in size and/or apparent material before lifting as well as their perceived weight afterwards. Their results showed that material exerted a stronger influence on weight expectations before lifting than size did. Note that these effects could be specific to their stimulus set, given that material differences appeared more pronounced than size differences (as shown illustratively in their Fig. 1 ). Nevertheless, they argued that conceptual expectancy explanations predict, in this case, that material should influence perception more strongly than size. However, contrary to what one might expect from these accounts, their results demonstrated that size influenced perception more strongly than material—despite the stronger predictive value of the latter on the expected weight.

These findings suggest that the SWI is a stronger illusion than the MWI, given that variations in size rather than surface material seem to produce larger and more reliable differences in perceived weight. This creates a dilemma for conceptual accounts of weight illusions, provided that size is not a greater predictor of weight than material. Whether or not the MWI is associated with smaller effect sizes than the SWI across the literature has never been systematically confirmed in a meta-analysis until now.

The second consideration relates to the role of different sensory input modalities in the SWI. Conceptual theories emphasise top-down influences driven by weight expectations that are based on an understanding of the relationship between an object’s weight and its other features. By this logic, the SWI should be consistent regardless of the particular sensory modality providing reliable conceptual information about the size of the stimuli. However, some studies report that the SWI is stronger when information about the size of the stimuli is obtained through the somatosensory system than when the same information is presented visually (Ellis & Lederman, 1993 ), posing another problem for conceptual expectancy accounts.

Ellis and Lederman ( 1993 ) directly compared the influence of different sensory modalities. In their study, the authors varied the availability of somatosensory and visual information. In one condition, participants were blindfolded and only received somatosensory information about the size of the stimuli by hefting them. In this case, haptic information was received through the pressure exerted from the objects on the touch receptors in the skin. Kinaesthetic information was also obtained via the proprioceptors in the muscles as the objects were balanced in the palm of each hand. In a second condition, participants could see the objects, but all somatosensory information about their size was removed by having the participants lift the stimuli using strings. In a third condition, participants were permitted to see the objects while they hefted them, providing the participants with information about the size of the objects via both somatosensory and visual channels. Comparisons between the three conditions indicated that the SWI was weakest when only visual information about size was available. The strength of the SWI was greater when somatosensory feedback about size was available. Namely, there was a greater difference in perceived weight across the objects when size information was gained through haptic and kinaesthetic feedback. Furthermore, the availability of visual information about size made no difference to illusion strength when somatosensory feedback was also available, a pattern also seen in Plaisier and Smeets’s ( 2015 ) Experiment 4.

The findings described in this section suggest that conceptual expectancies do not entirely explain the SWI. A systematic meta-analysis of these studies could shed light as to whether or not obtaining size information through somatosensory or visual modalities matter for the illusion. Next, we discuss alternative explanations that propose a role of bottom-up mechanisms.

Bottom-up processing

Other accounts of weight illusions emphasise the role of a third-party object feature in driving weight perception. These accounts propose that aside from mass, there is another variable that is processed or computed during handling that influences or is construed as perceived weight in a bottom-up fashion (see Fig. 1 ; J. Ross & Di Lollo, 1970 ; Stevens & Rubin, 1970 ). This process could be similar to the same way that a person might mistake flavour for taste. For example, the perception of a ‘hot’-tasting jalapeño does not arise from the taste buds, but rather from the pain receptors in the mouth and nose.

One of these accounts implicates physical density. This account is particularly relevant to the SWI because stimuli vary not only in size but also in density; the smaller of two objects of equal mass must also be denser. Although there are apparent or expected density differences in the MWI, there are no physical density differences in the MWI as there is in the SWI because MWI stimuli are identical in both size and mass. Accordingly, several research groups have considered how physical density may be implicated in the SWI (Chouinard et al., 2009 ; Harshfield & DeHardt, 1970 ; Peters, Ma, & Shams, 2016 ; H. E. Ross & Gregory, 1970 ; Stevens & Rubin, 1970 ; Thouless, 1931 ; Wolf, Bergmann Tiest, & Drewing, 2018 ). A number of SWI studies have examined how weight perception changes with density (J. Ross & Di Lollo, 1970 ; Stevens & Rubin, 1970 ; Wolf et al., 2018 ). One of these demonstrated that perceived weight can increase with density even when mass is held constant (J. Ross & Di Lollo, 1970 ).

Aside from density, other object features could be construed as weight. Some authors have emphasised how the processing of object properties that are relevant for action, or “affordances” (Gibson, 1979 ), might also influence weight perception. For example, Amazeen and Turvey ( 1996 ) demonstrated that perceived weight was predicted not only by an object’s mass but also by the distribution of its mass. They attached weights to different locations on long rods, which varied the rotational inertia of the rods as they were held and manipulated by their participants. The participants perceived the objects’ rotational inertia via the torques applied during the lift, which the authors argued was construed as a weight percept. Zhu and Bingham ( 2011 ) provide another study examining affordances in weight perception. Their results indicated that differences in perceived weight in the SWI were related to judgements of how far objects can be thrown, or what the authors referred to as their throwability affordance.

These processes could still occur alongside conceptual expectancy mechanisms (Buckingham, 2014 ). As discussed, conceptual expectancies influence weight perception in a top-down manner (see Fig. 1 ); for example, knowing that lead is heavy will cause one to expect a lead object to be heavy and consequently influence weight perception, as in the MWI. However, the processing of another feature can also influence weight perception in a bottom-up manner. For instance, lifting a denser object without considering its material composition will cause one to perceive it as being heavier if the brain misinterprets density as weight. Note that density is the determining factor that influences weight in both examples. However, it is the conceptual knowledge about an object’s expected or apparent density that influences weight perception in the first case, whereas it is the bottom-up processing of physical density in real time that gets translated or construed as weight in the second case. Thus, it is possible that both conceptual expectancies and bottom-up processing explain weight illusions. Considering both explanations could explain many findings that do not fit well with conceptual expectancy or bottom-up theories alone. An influence of bottom-up processing of physical density could account for a stronger SWI than MWI. However, bottom-up processing of density cannot explain the MWI because physical density is the same for both objects. Differences in density in the MWI are apparent, or expected, and thus differences are conceptual.

Unlike conceptual expectancy accounts, bottom-up explanations can allow for varying effects of sensory modality on the SWI given that sensory channels process information somewhat independently—at least in the earlier stages of sensory processing. Consider the ‘hot’ jalapeño analogy described earlier. A jalapeño feels excruciatingly more painful if it touches the eye than when it is inside the mouth. The same stimulus leads to two different levels of sensation when processed by these two independent channels. Compared with vision, information about an object’s weight is processed most directly by the somatosensory system via the proprioceptors in the muscles (McGlone & Reilly, 2010 ; Rowe, 2002 ). Vision can certainly extract an object’s weight, but only by observing how the limbs respond to an object’s weight during lifting (Buckingham, Michelakakis, & Cole, 2016b ; Buckingham et al., 2011b ), which is conceivably a more demanding cognitive process. Computing an object’s physical density requires information about both its size and its weight. In contrast to vision, the somatosensory system is designed to calculate both features more directly through haptic and kinaesthetic feedback (Ellis & Lederman, 1993 ). In this sense, the somatosensory system is arguably better placed to compute physical density with greater efficiency than the visual system is and could therefore potentially exert a larger effect on the SWI if bottom-up processes play a role. However, studies that have compared different sensory modalities are mixed and few in number. A systematic meta-analysis of SWI studies could shed light as to whether or not obtaining size information through somatosensory or visual modalities matters for the perceptual experience of the illusion.

The current study

We conducted a meta-analytic review of the SWI and MWI to assess support for (a) conceptual expectancy and (b) bottom-up processing explanations for weight illusions. We obtained descriptive data from studies in which subjective heaviness estimates were made for pairs or groups of objects that had the same mass and different volumes (SWI) or apparent material properties (MWI). Differences in reported heaviness between stimuli were combined to produce an effect size measure, which served as a common metric of illusion strength across studies. Other study details, including stimulus mass and volume, and the degree of visual and somatosensory access to the stimuli, were also recorded to quantify the contribution of these variables to the strength of the illusory weight experience.

We tested the following predictions. If conceptual expectancies entirely explain the SWI, then the SWI and MWI should be similar in strength, assuming they predict weight to a similar degree. Specifically, effect sizes for the two illusions across the literature base should have a comparable magnitude. Furthermore, size information obtained through somatosensory and visual channels should produce a comparable SWI. Alternatively, a contribution of bottom-up processing of physical density predicts a stronger SWI than MWI. Unlike conceptual expectancy accounts, bottom-up processing accounts allow for a variable effect of sensory modality on the SWI. A further prediction is that physical density should relate to illusion strength in the SWI. Namely, if the bottom-up processing of physical density contributes to forming a weight percept, then a greater difference in density across SWI objects should be associated with a greater difference in perceived heaviness and, therefore, a stronger SWI. Accordingly, the current study had the following aims:

Aim 1: To quantify and compare the strength of the SWI and MWI to determine if the type of concept matters. Addressing this aim will shed light into the viability of conceptual expectancy accounts.

Aim 2: To quantify the contribution of vision and somatosensory information about stimulus size in the SWI to determine if the type of sensory processing matters. Addressing this aim will shed light into the viability of bottom-up processing explanations.

Aim 3: To investigate the relationship between SWI strength and physical density differences across stimuli. Addressing this aim will shed light into the viability of a specific bottom-up processing explanation that is centred on the influence of density processing.

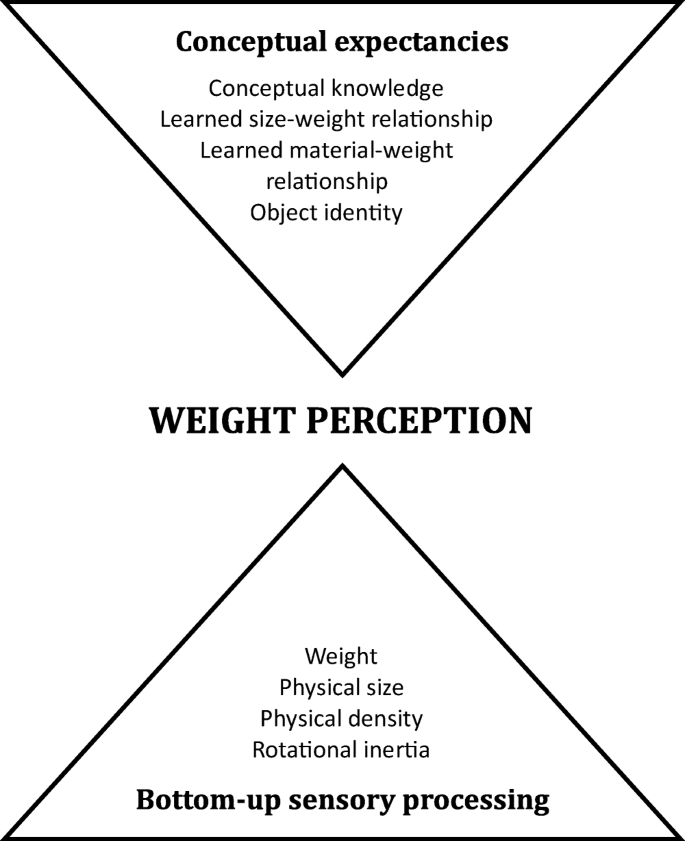
Sample of studies

The first author (E.S.) conducted literature searches in PubMed and Scopus for studies published before September 2017. Figure 2 provides a step-by-step illustrative account of how papers were searched and triaged by E.S. Using the search terms ‘size–weight illusion’ OR ‘material–weight illusion’, 179 published studies were found. Of these, 31 studies were excluded because they did not use stimuli that met criteria for either the SWI (same mass, different volume) or MWI (same mass, different apparent material). Twenty-four papers were excluded because they were review articles and/or did not report novel data (e.g., reanalysis or linguistic translation of previously published data). Nine studies were excluded because they did not employ a neurologically normal adult sample (e.g., children, neuropsychological cases with no control group). Thirty-eight papers were excluded because they did not include subjective, perceptual ratings of stimulus weight (e.g., participants gave a binary response indicating which one of two stimuli were heavier). A further 38 papers did not include sufficient information about experimental methodology, stimulus properties, or descriptive statistics to be included in the meta-analysis and were published prior to 2000, so it was deemed unlikely that the information could be obtained from the authors. For manuscripts with insufficient detail that were published in or after the year 2000, E.S. e-mailed authors for the additional information. Following this process, a further 16 studies were excluded because the required information could not be obtained. All authors who were contacted were also asked for any unpublished data sets or “file drawer” data. The final number of studies included was 28 (see Fig. 2 ). This sample included three unpublished data sets and 39 different experimental conditions.

Flow chart summarising search and screening processes for the meta-analysis study inclusion

Recorded/moderator variables

Details of the study design and stimulus properties from each experiment were recorded, including study N s, stimulus mass and volume, lifting style, degree of visual access, and experimental manipulations. To examine the effect of sensory input modality on illusion strength, the availability of visual and somatosensory information for each experimental condition was coded into the following dichotomous variables: Somatosensory (some, none); Vision (some, none), as detailed in Table 1 . Stimulus density was calculated by dividing mass by volume (g/cm 3 ).

Dependent measure

We recorded mean heaviness ratings for SWI or MWI objects (see Supplementary Material for the raw data). In most cases, these scores represented participants’ subjective magnitude estimates for two or more objects within an experiment or condition. In other cases, one of the objects was deemed the ‘standard’ and was allocated a certain heaviness value by the experimenter, against which participants gave a relative magnitude estimate of heaviness for the other object(s). If raw or standardised means and standard deviations ( SD s) were not reported in the manuscript, and could not be estimated precisely from graphs, then we contacted authors for the required information.

We used observations of effect size to represent the difference in perceived heaviness between pairs of illusory objects within an experiment. Cohen’s d was chosen as the measure of effect size because it is a standardised and easily interpretable measure of the difference between two means. Using a standardised index of effect size enabled us to compare illusion strength across studies without the original response scales influencing the results. Each observation of Cohen’s d was calculated as the difference between two mean heaviness ratings, divided by the pooled standard deviation (i.e., using the standard deviations of the two means, which represents variability across participants), weighted by sample size (Card, 2011 ; Cohen, 1988 ; Lipsey & Wilson, 2001 ). Data were organised such that there was one observation of d per stimulus pair. Given that most SWI or MWI studies include more than two stimuli, there was typically more than one observation of d per experiment. For example, a single SWI experiment with a small, medium, and large stimulus would yield three observations of d (i.e., small vs medium, small vs large, medium vs large).

The meta-analysis required us to quantify illusion strength across all included studies. To do so, we determined mean weighted d s and 95% confidence intervals (CI) for each illusion. First, we calculated a single, pooled effect size for each experiment, then used these pooled d s to determine the overall mean d s for the different illusions. We used the experiment-wise pooled d s to calculate the means to ensure that experiments including more than two stimuli (and therefore multiple observations of d ) were not overrepresented in the overall means. Pooled d s and their variances were calculated according to Borenstein, Hedges, Higgins, and Rothstein’s ( 2009 ) formulae for combining within-study effect sizes from multiple outcomes. Mean d s were weighted to account for sample size differences across experiments. The weighting procedure, including formulae for inverse variance weight values and SE Mean weighted d were taken from Card (2011; also see Lipsey & Wilson, 2001 ). This weighting procedure is commonly used in meta-analyses (e.g., Landry & Al-Taie, 2016 ). We interpreted values of d according to the following criteria: 0.2–0.49 = small effect size, 0.5–0.79 = medium effect size, and <0.8 = large effect size (Cohen, 1988 ).

To achieve Aim 1, mean weighted d s were calculated separately for the SWI and MWI. To achieve Aim 2, mean weighted d s were calculated independently for three versions of the SWI, according to the different levels of the vision and somatosensory variables as defined in Table 1 : (1) SWI-vision (vision: some; somatosensory: none); (2) SWI-somatosensory (vision: none; somatosensory: some); and (3) SWI-vision and somatosensory (vision: some; somatosensory: some). Thus, for the purpose of Aim 2, these three variations of the SWI were treated as independent illusions.

To achieve Aim 3, we investigated the relationship between SWI strength and physical density differences across stimuli. In investigating the effect of density differences, it was important to account for volume differences, which are inherent in the SWI and have been shown to vary with illusion strength (Ellis & Lederman, 1993 ; J. Ross & Di Lollo, 1970 ). Accordingly, we conducted a hierarchical regression analysis to examine the relationship between illusion strength and density differences, after controlling for the effects of volume. Note that the experiment-wise pooled d s could not be used as the dependent variable for this analysis because differences in volume and density across objects were averaged within the pooled d s. Accordingly, the dependent variable for this analysis comprised the original observations of d , which represent the difference in perceived weight for pairs of stimuli within a particular experiment or condition. For each observation of d , we calculated the difference in both volume and density across the object pair. The volume of the smaller object of the pair was expressed as a percentage of the larger one. Density differences were calculated by subtracting the density of the larger object from that of the smaller object. Note that stimulus volume (and therefore density) was unknown for a small number of objects (e.g., the trolleys in Schmidtler & Bengler’s, 2016 , experiments), as seen in Table 3 . These data points were excluded from this analysis. The supplementary material details the volume and density differences for all included object pairs and their observations of d .

Mean weighted d s for the different illusions are presented in Table 2 . Table 3 summarises all included experiments and their pooled d s, which were combined to produce the overall mean d s. Table 3 also contains the mean volume and density differences between SWI stimuli within each experiment. Forest plots displaying the pooled d s are presented separately for the MWI (Fig. 3 ) and each variant of the SWI (Fig. 4 ). The Supplementary Material contains detailed information about all collected observations of d , including the nature of the stimulus pair comprising each observation (e.g., small vs medium; medium vs large), as well as the mass, volume, density, and mean heaviness estimates for all stimuli.

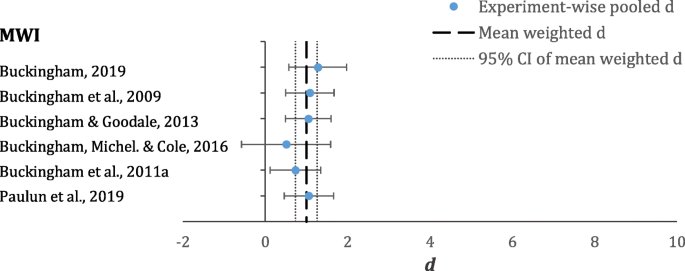
Forest plot displaying all experiment-wise pooled d s (circles) and mean weighted d (dashed line) and 95% confidence interval (CI; dotted line) for the mean weighted d for the MWI. Error bars represent 95% CIs for the experiment-wise pooled d s

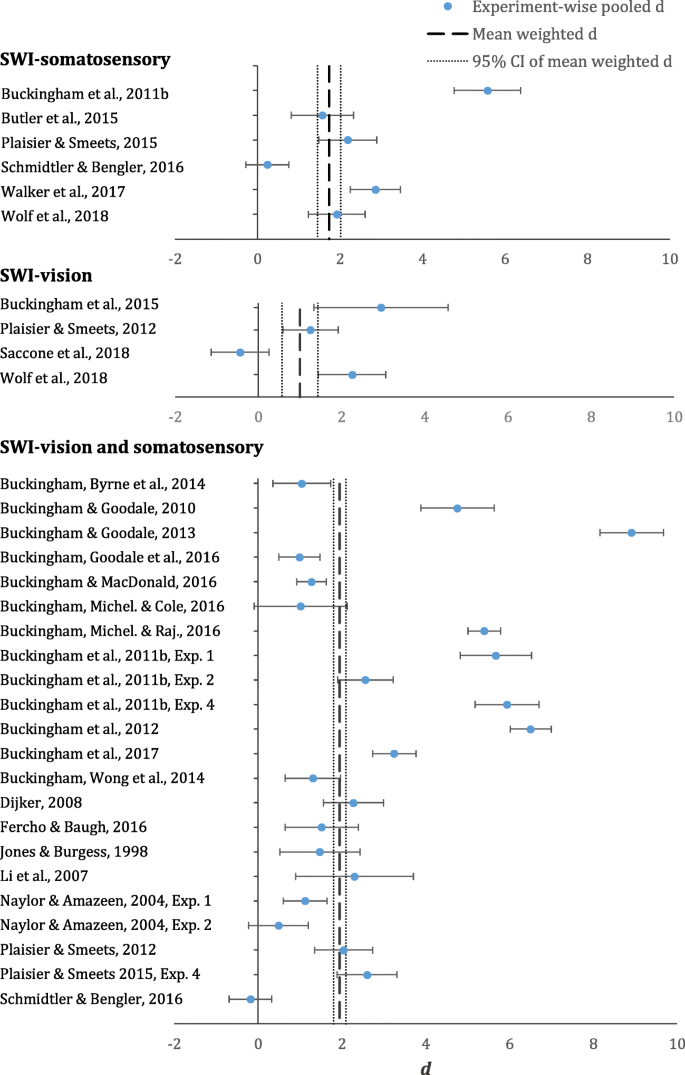
Forest plot displaying all experiment-wise pooled d s (circles), mean weighted d s (dashed lines) and 95% confidence interval (CI; dotted line) for the SWI-somatosensory (top), SWI-vision (middle) and SWI-vision and somatosensory (bottom). Error bars represent 95% CIs for the experiment-wise pooled d s

Aim 1: Overall effect of the SWI vs MWI

Mean weighted d s for the SWI and MWI are displayed in Table 2 . Both illusions have large effect sizes. Regarding the weighted means, there is no overlap in the 95% CIs, demonstrating a significant difference between the two means. Thus, the results demonstrate that the SWI is significantly stronger than the MWI.

Aim 2: Comparing sensory modalities in the SWI

Table 2 displays the mean weighted d s for the SWI-somatosensory, SWI-vision, and SWI-vision and somatosensory. All variants of the SWI have large mean effect sizes. Examination of the 95% CIs for the means reveal that the SWI- somatosensory and SWI-vision and somatosensory are comparable in strength, whereas the SWI-vision is weaker than the other two variants. Table 2 also displays the overall mean volume and density differences across stimuli for the three versions of the SWI. The 95% CIs for the mean volume and density differences demonstrate that the SWI groups are sufficiently matched in this respect. The three variants of the SWI are discussed in more detail below.

The mean weighted d for the SWI-somatosensory is 1.74 (95% CI [1.46, 2.02]). This mean estimate comprises pooled d s from six unique experimental conditions (see Table 3 and Fig. 4 ). The pooled d s for this variant of the SWI range from 0.24 (Schmidtler & Bengler, 2016 ) to 5.58 (Buckingham et al., 2011b ).

The mean weighted d for the SWI-vision is 1.00 (95% CI [0.57, 1.43]). This mean effect size comprises pooled d s from four unique experiments (see Table 3 and Fig. 4 ). The pooled d s for this variant of the SWI range from −0.44 (Saccone, Goldsmith, Buckingham, & Chouinard, 2018 ) to 2.95 (Buckingham, Milne, Byrne, & Goodale, 2015b ). As mentioned, the SWI-vision is a weaker illusion than the other two variants of the SWI. The upper boundary of the 95% CI for the SWI-vision is close to the lower boundary of the 95% CI for the SWI-somatosensory.

The mean weighted d for the SWI-vision and somatosensory is 1.95 (95% CI [1.80, 2.10]). This is the most common variant of the SWI. The mean effect size for this version of the SWI comprises pooled d s from 22 unique experimental conditions (see Table 3 and Fig. 4 ). The pooled d s range from values as low as −0.18 (Schmidtler & Bengler, 2016 ) and as high as 8.92 (Buckingham & Goodale, 2013 ).

Aim 3: Relationship between SWI strength and density difference

To address Aim 3, we examined the relationship between observations of d and physical density differences across SWI stimuli. First, two Pearson correlations were performed to test the independent relationships between SWI strength and both volume and density differences. The SWI was stronger when there was a greater volume discrepancy across the stimuli, r (140) = −.297, p < .001. A stronger SWI was also associated with greater differences in physical density, r (140) = .466, p < .001 (see Fig. 5 ).

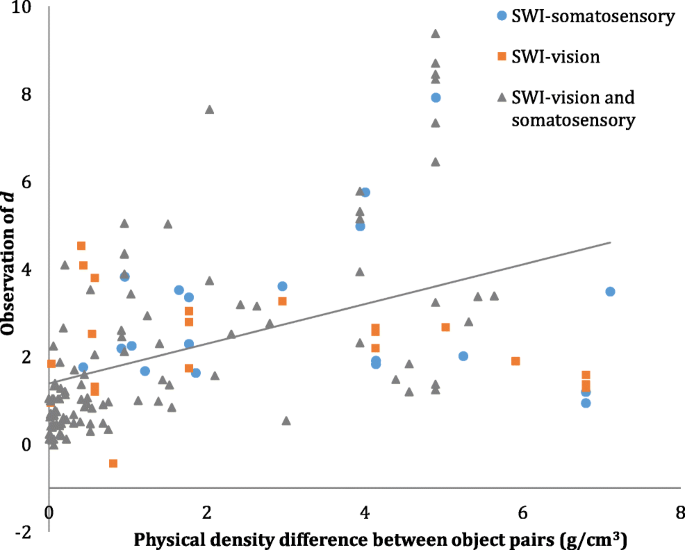
Scatterplot displaying the correlation, between observations of SWI effect sizes ( d ) and differences in density between test objects comprising that observation of d (g/cm3), r (140) = .466, p < .001. Blue circles depict observations of d from the SWI-somatosensory , orange squares depict observations of d from the SWI-vision , and grey triangles depict observations of d from the SWI-vision and somatosensory. The linear line of best fit is shown

Next, we performed a hierarchical regression analysis to test the relationship between SWI strength and density differences, while controlling for volume differences. Volume difference was entered in Step 1 of the analysis, R 2 = .088, F (1, 140) = 13.524, p < .001. Density difference was entered in Step 2, R 2 change = .135, F change (1, 140) = 24.147, p < .001. These results demonstrate that density differences across SWI stimuli explain 13.5% of the variance in d after controlling for differences in volume.

This study assessed support for (a) conceptual expectancies and (b) bottom-up processing explanations for weight illusions. To test predictions of these two types of accounts, the meta-analysis quantified and compared the strength of the SWI and MWI (Aim 1), as well as the influence of somatosensory and visual information about stimulus size on the SWI (Aim 2). As a further test of a contribution of bottom-up processing of physical density in the SWI, we also examined the relationship between SWI strength and physical density differences across stimuli (Aim 3). Overall, this meta-analysis provides support for both types of accounts.

Our first aim was to compare the SWI and MWI. The number of SWI experiments and the number of effect size observations we could calculate from them far exceeded those for the MWI. The results demonstrated a larger mean effect size for the SWI than the MWI, which supports preliminary claims in the existing literature (Buckingham, Bieńkiewicz, et al., 2015; Buckingham & Goodale, 2013 ; Vicovaro & Burigana, 2017 ). This finding is potentially consistent with the idea that conceptual expectancies do not entirely account for the SWI and could support a contribution of bottom-up processes to the illusion. However, we still observed a large mean effect size for the MWI. In this case, it is the expected but not the actual physical density that must account for the illusion – one that is based on a conceptual understanding of different materials and how this understanding is used to predict weight. Given that bottom-up processing of physical density cannot explain the MWI, this finding bolsters accounts based on conceptual expectancies.

We also note a higher degree of variability in the effect sizes for the SWI than for the MWI. The experiment-wise pooled d s for the MWI ranged between 0.51 and 1.28 (see Table 3 and Fig. 3 ), and these data points do not appear to deviate much from the weighted mean. Conversely, the pooled d s for the SWI were highly variable across studies, ranging from less than zero (Saccone et al., 2018 ; Schmidtler & Bengler, 2016 , in Table 3 and Fig. 4 ) to more than 8 (Buckingham & Goodale, 2013 , in Table 3 and Fig. 4). In addition, there appeared to be greater variability in sample sizes, methodologies, and experimental manipulations in the SWI than in the MWI, likely contributing to the greater variability in effect sizes for the SWI.

An important consideration, which we were unable to resolve, is that differences in effect sizes between the two illusions may have been driven by scalar differences for volume versus apparent material. To explain, discrepancies in volume between objects influence effect sizes for the SWI, whereas discrepancies in expected densities between objects influence effect sizes for the MWI. Could it be the case that the former is stronger in this meta-analysis because there were greater discrepancies in volume than expected density in the published literature? One could potentially resolve this by performing correlations between effect sizes and these discrepancies. For the MWI, one might expect to find a correlation between effect sizes and expected density differences, as informed by surface material. For example, if a polystyrene object is rated against an aluminium one, the effect size for the MWI should be larger than when the aluminium object is compared with wood because there is a greater difference in expected density in the former than the latter pair (see Ellis & Lederman, 1999 , for an example of this pattern). However, we did not have sufficient data points to test this possibility using a correlation analysis. Only four different surface materials have been employed (polystyrene, wood [oak], stone [granite], and aluminium; see Table S1 in the Supplementary Materials) in the MWI studies included in this meta-analysis, which is an insufficient number for fitting a correlation. In contrast, the SWI studies included in the meta-analysis varied considerably in volume differences (see Table S2 in the Supplementary Materials) and enabled us to perform a correlation between changes in size and weight perception. A comparison between these two correlations could have enabled us to establish if changes in size and material yielded similar or different changes in perceived weight.

The second aim of the meta-analysis was to examine the influence of somatosensory and visual information about stimulus size on the SWI. All three groupings of studies (i.e., SWI-vision and somatosensory, SWI-somatosensory, and SWI-vision) had large mean effect sizes. Thus, our findings suggest that the SWI is robust regardless of which sensory modality processes size information. The 95% CI for the SWI-vision appears greater than the other two variants, perhaps because there were fewer pooled effect sizes for the SWI-vision. In addition, the SWI-vision and somatosensory group was considerably more prevalent in the literature than either of the other two groups. The SWI-vision and somatosensory group also appeared to have the most variability in the pooled effect sizes (see Fig. 4 ) and the nature of the experiments performed (see Table 3 ). For example, Schmidtler and Bengler ( 2016 ) had participants push heavy stimuli atop a large trolley and had them rate ‘subjective strain’ as an indicator of perceived heaviness. Buckingham, Wong, Tang, Gribble, and Goodale ( 2014b ) had participants witness erroneous or inappropriate lifts of the SWI stimuli before they lifted the stimuli themselves. These are unique SWI experiments whereas experiments in studies using SWI-somatosensory and SWI-vision designs tended to be more typical (see Table 3 ). This is expected for a greater number of studies within a particular group of studies given that novelty is expected in publications. A greater range of experiments may have contributed to the increased variability in effect sizes seen in the SWI-vision and somatosensory group.

Comparing the effects between sensory modalities on illusion strength yielded results that were consistent with preliminary claims from the existing literature. The mean effect sizes for the SWI-somatosensory and SWI-vision and somatosensory groups were comparable in strength. This finding accords with past research showing that the SWI is consistent in strength regardless of visual access to the stimuli when somatosensory feedback about size is available (Ellis & Lederman, 1993 ; Plaisier & Smeets, 2015 ). We also demonstrated that the SWI-vision is weaker than the other SWI variants, which supports previous findings regarding sensory input modality by Ellis and Lederman ( 1993 ). These results are inconsistent with conceptual accounts, suggesting that conceptual expectancies do not entirely explain the SWI. Instead, the findings support an influence of bottom-up processing mechanisms on the illusion. Importantly, variations in volume and density were matched across these groups. Therefore, we can rule out the possibility that differences in effect sizes between SWI variants were driven by differences in either volume or density.

Our third aim was to examine the relationship between physical density and the SWI. The meta-analysis revealed a strong, positive correlation between illusion strength and physical density differences between stimuli, which supports past research implicating density in the SWI (Chouinard et al., 2009 ; J. Ross & Di Lollo, 1970 ; Stevens & Rubin, 1970 ; Wolf et al., 2018 ). Our results demonstrated that the SWI is stronger when there is a greater difference in density between the test objects, and that this relationship persists after statistically controlling for volume differences. Specifically, our hierarchical regression analysis revealed that this relationship was weaker after accounting for volume, suggesting that the effect of density in the SWI occurs partly but not entirely through the processing of physical size. These findings demonstrate that the postlift processing of density contributes to the SWI and that physical density contributes to our percept of weight more generally.

The findings of Aims 2 and 3 paint an interesting picture of how bottom-up processing of physical density might influence perception in the SWI. The results indicate that density contributes to the illusion and that size exerts a stronger influence on perceived weight when processed through somatosensory channels. Although visually derived size information can be used to compute density (e.g., Wolf et al., 2018 ), as we proposed earlier, the somatosensory system may perform this computation more efficiently because it is designed to calculate both size and weight more directly through haptic and kinaesthetic feedback (Ellis & Lederman, 1993 ; McGlone & Reilly, 2010 ; Rowe, 2002 ). Our results suggest that when both size and weight are processed through somatosensory channels, density is computed in a way that is more likely to influence perceived weight.

Our results also suggest the SWI-vision is comparable in strength to the MWI, whereas the other two SWI variants are significantly stronger. In this meta-analysis, information about size and material were not obtained through the somatosensory system in the SWI-vision and MWI studies, respectively. Thus, this finding could mean that a conceptual expectancies mechanism influences perception comparably when either size or material informs expectations, but only when the somatosensory system is not providing size information that is used to compute physical density. Therefore, density, when computed via somatosensory channels, could be an important contributing factor as to why the SWI is stronger than other weight illusions, including the MWI and presumably other weight illusions in which the only varied feature is a conceptual one.

Of note, Ellis and Lederman ( 1999 ) showed that the MWI can be elicited in blindfolded participants when the materials of the objects are felt by haptic exploration but are not seen. However, it is still possible that the illusion in this instance is driven by top-down mechanisms. Consider being blindfolded and handling objects made from the various materials that are typically employed in the MWI, such as wood, polystyrene, and metal. Their tactile sensation can invoke visual images of these materials. This is evident from neuroimaging research demonstrating that the same areas in the visual cortex that are engaged in their visual presentation are also engaged when participants explore these materials by touch only (Podrebarac, Goodale, & Snow, 2014 ). This can only be explained by top-down mechanisms given that there are no known afferent projections from the spinal cord to the visual cortex.

Another consideration that we could not address directly in the current study is the potential for publication bias. This is particularly relevant for our comparison of the SWI and MWI because the number of published SWI experiments greatly exceeds that of the MWI. The current study included 32 experiment-wise pooled effect sizes for the SWI, but only six for the MWI. This disparity could suggest that unpublished null results or ‘file-drawer’ data exist for the MWI. If this were the case, our mean estimate for the MWI could be inflated. In this vein, it could be relevant that the demonstrated difference between the two illusions was not as great as previous studies suggested (e.g., SWI, η p 2 = .98 vs MWI, η p 2 = .42; Buckingham & Goodale, 2013 ). In his qualitative review, Buckingham estimated the SWI to be around three times as strong as the MWI, which does not accord with our findings. As mentioned earlier, we attempted to uncover file-drawer data in our correspondence with authors. Beyond this, we were unable to address publication bias statistically owing to the small number of published MWI studies.

We also note that there is a remaining theoretical issue regarding the comparison between the SWI and MWI. As this is the first meta-analysis to quantify and compare effect sizes for the two illusions as documented in the literature, we provide preliminary evidence that the SWI has larger effect sizes than the MWI. That is, variations in physical size result in larger differences in perceived weight than apparent material in weight illusion paradigms. This finding, considered alongside results from Aims 2 and 3 of our meta-analysis, informs on the relative contributions of conceptual expectancies and bottom-up processing of physical density in weight perception. However, as discussed above, it is still unknown whether proportional changes in the relevant defining variables for the SWI (i.e., volume) and the MWI (i.e., expected density based on apparent material) produce comparable changes in perceived weight. Further investigation into the MWI is required, given the paucity of studies on this illusion relative to the SWI. We suggest that the MWI is examined further using a greater range of materials. Only then can we fully understand how changes in material influence weight perception and whether or not this follows a similar scale as changes in size.

Overall, this meta-analysis demonstrates that that both conceptual expectancies and bottom-up processing of physical density contribute to the SWI. We demonstrated that the MWI has large effect sizes, consistent with conceptual expectancies accounts of weight illusions. However, the finding of an even stronger SWI, as well as the relationship between SWI strength and physical density differences, supports a contribution of density processing via the somatosensory system.

Last, not all individual findings in the literature are well explained by the conclusions drawn from this meta-analysis. For example, Plaisier and Smeets ( 2015 ) demonstrated an influence of physical size on perceived weight that was independent from volume of matter (which should inform conceptual expectancies) and physical density. It is expected that some studies will not accord with our meta-analysis. This does not negate their validity, nor does it negate the validity of our study. There are a number of possible reasons for disagreement. Meta-analyses aim to summarise and synthesise the existing literature as a whole, which in the process dilutes the effect of individual studies and their particular task and contextual constraints.

Amazeen, E. L., & Turvey, M. T. (1996). Weight perception and the haptic size–weight illusion are functions of the inertia tensor. Journal of Experimental Psychology: Human Perception and Performance, 22 (1), 213–232. https://doi.org/10.1037//0096-1523.22.1.213

Article   PubMed   Google Scholar  

Baugh, L. A., Kao, M., Johansson, R. S., & Flanagan, J. R. (2012). Material evidence: Interaction of well-learned priors and sensorimotor memory when lifting objects. Journal of Neurophysiology, 108 (5), 1262–1269. https://doi.org/10.1152/jn.00263.2012

Borenstein, M., Hedges, L. V., Higgins, J. P., & Rothstein, H. R. (2009). Introduction to meta-analysis . Chichester, UK: John Wiley & Sons.

Book   Google Scholar  

Buckingham, G. (2014). Getting a grip on heaviness perception: A review of weight illusions and their probable causes. Experimental Brain Research, 232 (6), 1623–1629. https://doi.org/10.1007/s00221-014-3926-9

Buckingham, G. (2019). Unpublished m aterial-weight illusion dataset . Unpublished raw data. https://doi.org/10.17605/OSF.IO/3VDYN

Buckingham, G., Bieńkiewicz, M., Rohrbach, N., & Hermsdörfer, J. (2015a). The impact of unilateral brain damage on weight perception, sensorimotor anticipation, and fingertip force adaptation. Vision Research, 115 , 231–237. https://doi.org/10.1016/j.visres.2015.02.005

Buckingham, G., Byrne, C. M., Paciocco, J., van Eimeren, L., & Goodale, M. A. (2014a). Weightlifting exercise and the size–weight illusion. Attention, Perception, & Psychophysics, 76 (2), 452–459. https://doi.org/10.3758/s13414-013-0597-8

Article   Google Scholar  

Buckingham, G., Cant, J. S., & Goodale, M. A. (2009). Living in a material world: How visual cues to material properties affect the way that we lift objects and perceive their weight. Journal of Neurophysiology, 102 (6), 3111–3118. https://doi.org/10.1152/jn.00515.2009

Buckingham, G., & Goodale, M. A. (2010). Lifting without seeing: The role of vision in perceiving and acting upon the size weight illusion. PLOS ONE, 5 (3). https://doi.org/10.1371/journal.pone.0009709

Buckingham, G., & Goodale, M. A. (2013). Size matters: A single representation underlies our perceptions of heaviness in the size–weight illusion. PLOS ONE, 8 (1). https://doi.org/10.1371/journal.pone.0054709

Buckingham, G., Goodale, M. A., White, J. A., & Westwood, D. A. (2016a). Equal-magnitude size–weight illusions experienced within and between object categories. Journal of Vision, 16 (3), 1–9. https://doi.org/10.1167/16.3.25

Buckingham, G., & MacDonald, A. (2016). The weight of expectation: Implicit, rather than explicit, prior expectations drive the size–weight illusion. The Quarterly Journal of Experimental Psychology, 69 (9), 1831–1841. https://doi.org/10.1080/17470218.2015.1100642

Buckingham, G., Michelakakis, E. E., & Cole, J. (2016b). Perceiving and acting upon weight illusions in the absence of somatosensory information. Journal of Neurophysiology, 115 (4), 1946–1953. https://doi.org/10.1152/jn.00318.2015

Article   PubMed   PubMed Central   Google Scholar  

Buckingham, G., Michelakakis, E. E., & Rajendran, G. (2016c). The influence of prior knowledge on perception and action: Relationships to autistic traits. Journal of Autism and Developmental Disorders, 46 (5), 1716–1724. https://doi.org/10.1007/s10803-016-2701-0

Buckingham, G., Milne, J. L., Byrne, C. M., & Goodale, M. A. (2015b). The size–weight illusion induced through human echolocation. Psychological Science, 26 (2), 237–242. https://doi.org/10.1177/0956797614561267

Buckingham, G., Ranger, N. S., & Goodale, M. A. (2011a). The material–weight illusion induced by expectations alone. Attention, Perception, & Psychophysics, 73 (1), 36–41. https://doi.org/10.3758/s13414-010-0007-4

Buckingham, G., Ranger, N. S., & Goodale, M. A. (2011b). The role of vision in detecting and correcting fingertip force errors during object lifting. Journal of Vision, 11 (1), 4–4. https://doi.org/10.1167/11.1.4

Buckingham, G., Ranger, N. S., & Goodale, M. A. (2012). Handedness, laterality and the size-weight illusion. Cortex, 48 (10), 1342–1350. https://doi.org/10.1016/j.cortex.2011.09.007

Buckingham, G., Reid, D., & Potter, L. M. (2018). How prior expectations influence older adults’ perception and action during object interaction. Multisensory Research, 31 (3/4), 301–316. https://doi.org/10.1163/22134808-00002585

Buckingham, G., Wong, J. D., Tang, M., Gribble, P. L., & Goodale, M. A. (2014b). Observing object lifting errors modulates cortico-spinal excitability and improves object lifting performance. Cortex, 50 , 115–124. https://doi.org/10.1016/j.cortex.2013.07.004

Butler, A. A., Héroux, M. E., & Gandevia, S. C. (2015). How weight affects the perceived spacing between the thumb and fingers during grasping. PLOS ONE, 10 (5). https://doi.org/10.1371/journal.pone.0127983

Card, N. A. (2011). Applied meta-analysis for social science research. New York, NY: Guilford Press.

Google Scholar  

Chang, E. C., Flanagan, J. R., & Goodale, M. A. (2008). The intermanual transfer of anticipatory force control in precision grip lifting is not influenced by the perception of weight. Experimental Brain Research, 185 (2), 319–329. https://doi.org/10.1007/s00221-007-1156-0

Charpentier, A. (1886). Sur les sensations de poids (note présentée par M. D’Arsonval le 3 avril)[On sensations of weight (note presented by Mr. D’Arsonval on the 3rd of April)]. Comptes Rendus Hebdomadaires des Séances et Mémoires de la Société de Biologie, 38 , 169–170.

Charpentier, A. (1891). Experimental analysis of some elements of the feeling of weight (Analyse experimentale de quelgues elements de la sensation de poids). Archives de Physiologie Normale et Pathologique, 3 , 122–135.

Chouinard, P. A., Large, M. E., Chang, E. C., & Goodale, M. A. (2009). Dissociable neural mechanisms for determining the perceived heaviness of objects and the predicted weight of objects during lifting: An fMRI investigation of the size-weight illusion. NeuroImage, 44 (1), 200–212. https://doi.org/10.1016/j.neuroimage.2008.08.023

Cohen, J. (1988). Statistical power analysis for the behavioral sciences. New York, NY: Routledge Academic.

De Camp, J. (1917). The influence of color on apparent weight: A preliminary study. Journal of Experimental Psychology, 2 (5), 347. https://doi.org/10.1037/h0075903

Dijker, A. J. M. (2008). Why Barbie feels heavier than Ken: The influence of size-based expectancies and social cues on the illusory perception of weight. Cognition, 106 (3), 1109–1125. https://doi.org/10.1016/j.cognition.2007.05.009

Dijker, A. J. M. (2014). The role of expectancies in the size-weight illusion: A review of theoretical and empirical arguments and a new explanation. Psychonomic Bulletin & Review, 21 (6), 1404–1414. https://doi.org/10.3758/s13423-014-0634-1

Dresslar, F. B. (1894). Studies in the psychology of touch. The American Journal of Psychology, 6 (3), 313–368. https://doi.org/10.2307/1411644

Ellis, R. R., & Lederman, S. J. (1993). The role of haptic versus visual volume cues in the size–weight illusion. Perception & Psychophysics, 53 (3), 315–324. https://doi.org/10.3758/BF03205186

Ellis, R. R., & Lederman, S. J. (1998). The golf-ball illusion: evidence for top-down processing in weight perception. Perception, 27 (2), 193-201. https://doi.org/10.1068/p270193

Ellis, R. R., & Lederman, S. J. (1999). The material-weight illusion revisited. Perception & Psychophysics, 61 (8), 1564–1576. https://doi.org/10.3758/bf03213118

Fercho, K., & Baugh, L. A. (2016). Cognitive attribution of the source of an error in object-lifting results in differences in motor generalization. Experimental Brain Research, 234 (9), 2667–2676. https://doi.org/10.1007/s00221-016-4670-0

Flanagan, J. R., & Beltzner, M. A. (2000). Independence of perceptual and sensorimotor predictions in the size–weight illusion. Nature Neuroscience, 3 (7), 737–741. https://doi.org/10.1038/76701

Flanagan, J. R., Bittner, J. P., & Johansson, R. S. (2008). Experience can change distinct size–weight priors engaged in lifting objects and judging their weights. Current Biology, 18 (22), 1742–1747. https://doi.org/10.1016/j.cub.2008.09.042

Gibson, J. J. (1979). The ecological approach to visual perception. Boston, MA: Houghton Mifflin.

Grandy, M. S., & Westwood, D. A. (2006). Opposite perceptual and sensorimotor responses to a size–weight illusion. Journal of Neurophysiology, 95 (6), 3887–3892. https://doi.org/10.1152/jn.00851.2005

Harshfield, S. P., & DeHardt, D. C. (1970). Weight judgment as a function of apparent density of objects. Psychonomic Science, 20 (6), 365–366. https://doi.org/10.3758/bf03335692

Jones, L. F., & Burgess, P. R. (1998). Neural gain changes subserving perceptual acuity. Somatosensory and Motor Research, 15 (3), 190–199. https://doi.org/10.1080/08990229870754

Kahrimanovic, M., Bergmann Tiest, W. M., & Kappers, A. (2011). Characterization of the haptic shape–weight illusion with 3D objects. IEEE Transactions on haptics, 4 (4), 316–320. https://doi.org/10.1109/TOH.2011.22

Landry, O., & Al-Taie, S. (2016). A meta-analysis of the Wisconsin Card Sort Task in autism. Journal of Autism and Developmental Disorders, 46 (4), 1220–1235. https://doi.org/10.1007/s10803-015-2659-3

Li, Y., Randerath, J., Goldenberg, G., & Hermsdörfer, J. (2007). Grip forces isolated from knowledge about object properties following a left parietal lesion. Neuroscience Letters, 426 (3), 187–191. https://doi.org/10.1016/j.neulet.2007.09.008

Lipsey, M. W., & Wilson, D. B. (2001). Practical meta-analysis. Thousand Oaks, CA: SAGE Publications.

McGlone, F., & Reilly, D. (2010). The cutaneous sensory system. Neuroscience & Biobehavioral Reviews, 34 (2), 148–159. https://doi.org/10.1016/j.neubiorev.2009.08.004

Naylor, Y. K., & Amazeen, E. L. (2004). The size–weight illusion in team lifting. Human Factors, 46 (2), 349–356. https://doi.org/10.1518/hfes.46.2.349.37336

Paulun, V. C., Buckingham, G., Goodale, M. A., & Fleming, R. W. (2019). The material–weight illusion disappears or inverts in objects made of two materials. Journal of Neurophysiology . https://doi.org/10.1152/jn.00199.2018

Peters, M. A. K., Ma, W. J., & Shams, L. (2016). The size–weight illusion is not anti-Bayesian after all: A unifying Bayesian account. PeerJ, 2016 (6). https://doi.org/10.7717/peerj.2124

Plaisier, M. A., & Smeets, J. B. J. (2012). Mass is all that matters in the size–weight illusion. PLOS ONE, 7 (8). https://doi.org/10.1371/journal.pone.0042518

Plaisier, M. A., & Smeets, J. B. J. (2015). Object size can influence perceived weight independent of visual estimates of the volume of material. Scientific Reports, 5 . https://doi.org/10.1038/srep17719

Podrebarac, S. K., Goodale, M. A., & Snow, J. C. (2014). Are visual texture-selective areas recruited during haptic texture discrimination? NeuroImage, 94 , 129–137. https://doi.org/10.1016/j.neuroimage.2014.03.013

Ross, H. E. (1969). When is a weight not illusory? The Quarterly Journal of Experimental Psychology, 21 (4), 346–355.

Ross, H. E., & Gregory, R. (1970). Weight illusions and weight discrimination—A revised hypothesis. The Quarterly Journal of Experimental Psychology, 22 (2), 318–328. https://doi.org/10.1080/00335557043000267

Ross, J., & Di Lollo, V. (1970). Differences in heaviness in relation to density and weight. Perception & Psychophysics, 7 (3), 161–162. https://doi.org/10.3758/bf03208648

Rowe, M. J. (2002). The synaptic linkage for tactile and kinaesthetic inputs to the dorsal column nuclei. In S. C. Gandevia, U. Proske, & D. G. Stuart (Eds.), Sensorimotor control of movement and posture (pp. 47–55). Boston, MA: Springer US.

Chapter   Google Scholar  

Saccone, E. J., & Chouinard, P. A. (2019). The influence of size in weight illusions is unique relative to other object features. Psychonomic Bulletin & Review , 26(1), 77–89. https://doi.org/10.3758/s13423-018-1519-5

Saccone, E. J., Goldsmith, R. M., Buckingham, G., & Chouinard, P. A. (2018). Contrasting the effects of size and liquid volume content on the perceived weight of objects.  Unpublished manuscript.

Schmidtler, J., & Bengler, K. (2016). Size–weight illusion in human–robot collaboration. Paper presented at the 25th IEEE International Symposium onRobot and Human Interactive Communication (RO-MAN), New York, NY, USA.

Seashore, C. E. (1899). Some psychological statistics II. The material weight illusion. University of Iowa Studies in Psychology, 2 , 36–46.

Stevens, J. C., & Rubin, L. L. (1970). Psychophysical scales of apparent heaviness and the size–weight illusion. Perception & Psychophysics, 8 (4), 225–230. https://doi.org/10.3758/BF03210210

Thouless, R. H. (1931). Phenomenal regression to the ‘real’object, II. British Journal of Psychology, 22 (1), 1–30.

Vicovaro, M., & Burigana, L. (2017). Contribution of surface material and size to the expected versus the perceived weight of objects. Attention, Perception, & Psychophysics, 79 (1), 306–319. https://doi.org/10.3758/s13414-016-1212-6

Walker, P., Francis, B. J., & Walker, L. (2010). The brightness-weight illusion: Darker objects look heavier but feel lighter. Experimental Psychology, 57 (6), 462–469. https://doi.org/10.1027/1618-3169/a000057

Walker, P., Scallon, G., & Francis, B. (2017). Cross-sensory correspondences: Heaviness is dark and low-pitched. Perception, 46 (7), 772–792. https://doi.org/10.1177/0301006616684369

Wolf, C., Bergmann Tiest, W. M., & Drewing, K. (2018). A mass-density model can account for the size–weight illusion. PLOS ONE, 13 (2), e0190624. https://doi.org/10.1371/journal.pone.0190624

Wolfe, H. K. (1898). Some effects of size on judgments of weight. Psychological Review, 5 (1), 25–54. https://doi.org/10.1037/h0073342

Zhu, Q., & Bingham, G. P. (2011). Human readiness to throw: The size-weight illusion is not an illusion when picking the best objects to throw. Evolution and Human Behavior, 32 (4), 288–293. https://doi.org/10.1016/j.evolhumbehav.2010.11.005

Download references

Acknowledgements

This work was supported by the Australian Research Council (DP170103189). We have no conflicts of interest to declare.

Author information

Authors and affiliations.

School of Psychology and Public Health, La Trobe University, Edwards Road, Flora Hill, Victoria, 3552, Australia

Elizabeth J. Saccone, Oriane Landry & Philippe A. Chouinard

You can also search for this author in PubMed   Google Scholar

Corresponding author

Correspondence to Elizabeth J. Saccone .

Additional information

Publisher’s note.

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Electronic supplementary material

(DOCX 56 kb)

(DOCX 249 kb)

(XLSX 44 kb)

Rights and permissions

Reprints and permissions

About this article

Saccone, E.J., Landry, O. & Chouinard, P.A. A meta-analysis of the size-weight and material-weight illusions. Psychon Bull Rev 26 , 1195–1212 (2019). https://doi.org/10.3758/s13423-019-01604-x

Download citation

Published : 01 May 2019

Issue Date : 15 August 2019

DOI : https://doi.org/10.3758/s13423-019-01604-x

Share this article

Anyone you share the following link with will be able to read this content:

Sorry, a shareable link is not currently available for this article.

Provided by the Springer Nature SharedIt content-sharing initiative

• Size-Weight illusion
• Material-Weight illusion
• Weight perception
• Find a journal
• Publish with us
• Track your research

• Subscriber Services
• For Authors
• Publications
• Archaeology
• Art & Architecture
• Bilingual dictionaries
• Classical studies
• Encyclopedias
• English Dictionaries and Thesauri
• Language reference
• Linguistics
• Media studies
• Medicine and health
• Names studies
• Performing arts
• Science and technology
• Social sciences
• Society and culture
• Overview Pages
• Subject Reference
• English Dictionaries
• Bilingual Dictionaries

Recently viewed (0)

• Save Search
• Share This Facebook LinkedIn Twitter

Related Content

Size–weight illusion, quick reference.

A powerful cognitive illusion that causes approximately 98 per cent of people to judge an object to be heavier than another object of the same weight but much larger size when the two are lifted by hand. In a simple home demonstration of the illusion, pieces of lead or other heavy material may be placed in two different-sized containers and surrounded by sand to prevent them from moving about and from being visible if the containers are transparent, and the weights of the containers may be adjusted until they are identical, whereupon the smaller container will feel much heavier than the larger one. In a classic experiment on this illusion, 100 US military officers judged a smaller object to be on average two and a half times as heavy as one that was the same weight but twice the size in each dimension. The illusion was first reported in 1889 by the German psychologists Georg Elias Müller (1850–1934) and Friedrich Schumann (1863–1940). It is sometimes classified as a tactile illusion. Also called Charpentier's illusion .

From:   size-weight illusion   in  A Dictionary of Psychology »

Related content in Oxford Reference

Reference entries.

View all related items in Oxford Reference »

Search for: 'size–weight illusion' in Oxford Reference »

• Oxford University Press

PRINTED FROM OXFORD REFERENCE (www.oxfordreference.com). (c) Copyright Oxford University Press, 2023. All Rights Reserved. Under the terms of the licence agreement, an individual user may print out a PDF of a single entry from a reference work in OR for personal use (for details see Privacy Policy and Legal Notice ).

date: 19 December 2024

• Cookie Policy
• Privacy Policy
• Legal Notice
• Accessibility
• [66.249.64.20|162.248.224.4]
• 162.248.224.4

Character limit 500 /500

Charpentier (1891) on the size-weight illusion

Affiliation.

• 1 Department of Psychology, Queen's University, Kingston, ON, Canada. [email protected]
• PMID: 10598479
• DOI: 10.3758/bf03213127

This paper offers background for an English translation of an article originally published in 1891 by Augustin Charpentier (1852-1916), as well as a summary of it. The article is frequently described as providing the first experimental evidence for the size-weight illusion. A comparison of experiments on the judged heaviness of lifted weights carried out by Weber (1834) and by Charpentier (1891) supports the view that Charpentier's work deserves priority; review of other experimental studies on the size-weight illusion in the 1890s suggests that the idea that the illusion depended on "disappointed expectations," especially with respect to speed of lift, became dominant almost immediately following the publication of Charpentier's paper. The fate of this and other ideas, including "motor energy," in 20th-century research on the illusion is briefly described.

Publication types

• Historical Article
• Research Support, Non-U.S. Gov't
• History, 19th Century
• History, 20th Century
• Psychophysics / history*
• Size Perception*
• Weight Perception*

Personal name as subject

• A Charpentier

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

• View all journals
• My Account Login
• Explore content
• About the journal
• Publish with us
• Sign up for alerts
• Open access
• Published: 23 March 2021

The size-weight illusion is unimpaired in individuals with a history of congenital visual deprivation

• Rashi Pant 1 ,
• Maria J. S. Guerreiro 1 ,
• Pia Ley 1 ,
• Davide Bottari 1 , 3 ,
• Idris Shareef 2 ,
• Ramesh Kekunnaya   ORCID: orcid.org/0000-0001-5789-2300 2 &
• Brigitte Röder 1  

Scientific Reports volume  11 , Article number:  6693 ( 2021 ) Cite this article

3044 Accesses

10 Citations

3 Altmetric

Metrics details

• Cognitive neuroscience
• Visual system

Visual deprivation in childhood can lead to lifelong impairments in multisensory processing. Here, the Size-Weight Illusion (SWI) was used to test whether visuo-haptic integration recovers after early visual deprivation. Normally sighted individuals perceive larger objects to be lighter than smaller objects of the same weight. In Experiment 1, individuals treated for dense bilateral congenital cataracts (who had no patterned visual experience at birth), individuals treated for developmental cataracts (who had patterned visual experience at birth, but were visually impaired), congenitally blind individuals and normally sighted individuals had to rate the weight of manually explored cubes that differed in size (Small, Medium, Large) across two possible weights (350 g, 700 g). In Experiment 2, individuals treated for dense bilateral congenital cataracts were compared to sighted individuals in a similar task using a string set-up, which removed haptic size cues. In both experiments, indistinguishable SWI effects were observed across all groups. These results provide evidence that early aberrant vision does not interfere with the development of the SWI, and suggest a recovery of the integration of size and weight cues provided by the visual and haptic modality.

Similar content being viewed by others

Visual experience shapes the Bouba-Kiki effect and the size-weight illusion upon sight restoration from congenital blindness

Early visual deprivation disrupts the mental representation of numbers in visually impaired children

The relationship between reflex eye realignment and the percept of single vision in young children

Introduction.

Infants born with dense bilateral cataracts lack patterned vision until their sight is restored by cataract removal surgery. When surgery is performed late, i.e. beyond the first few weeks from birth, these individuals have been reported to suffer from permanent visual and multisensory impairments 1 , 2 , 3 , 4 , 5 . These impairments are hypothesized to be a behavioral consequence of neural system changes, resulting from aberrant sensory input within a sensitive period of development 6 , 7 . One way to assess visual and multisensory functional recovery in cataract reversal individuals is to test their susceptibility to well-known perceptual illusions. Perceptual illusions are typically extremely robust, suggesting that they arise from automatic processing principles 8 . Thus, the lack (or reduced likelihood) of perceiving a visual or multisensory illusion is indicative of impaired visual or multisensory processing, respectively.

Putzar et al. 9 tested the automatic detection of illusory contours in congenital cataract reversal individuals who had undergone cataract surgery between 1 and 17 months of age. They observed deficits in individuals who experienced more than 5–6 months of visual deprivation, and interpreted this result as evidence for impairments in the automatic binding of visual features—i.e. visual feature binding. This finding was extended by McKyton et al. 10 to a population of cataract reversal individuals who had undergone surgery only after the age of 5 years. These results suggested that early visual experience is required to acquire the neural circuits necessary for visual feature binding, providing evidence in favor of the sensitive period hypothesis. While this conclusion is compatible with the long developmental trajectory of illusory contour perception 11 , other studies replicated this finding for individuals treated for monocular 12 (but not binocular) congenital cataracts.

Gandhi et al. tested the Ponzo and Müller-Lyer illusions, wherein equally long lines are perceived to be of different lengths depending on their surroundings 13 . Forty-eight hours after cataract surgery, they found a full recovery of this illusion 13 , despite the fact that sight was partially restored only at 8 years of age or later. Since both these illusions were thought to arise from the interpretation of two-dimensional perspective cues as three-dimensional depth, they concluded that this process did not depend on early childhood vision.

Illusions have additionally been used to investigate the extent of multisensory recovery following cataract surgery. In an initial study, Putzar et al. 14 employed an audio-visual temporal capture effect, characterized as follows: if an auditory stimulus is presented with a short temporal offset with respect to a visual stimulus, the visual stimulus is often perceived as temporally shifted towards the time point when the sound was presented. This effect was significantly reduced in congenital cataract reversal individuals 14 . The residual visual impairments would have, according to the inverse efficiency rule of multisensory integration, predicted a larger capture in this group, suggesting that the multisensory binding process was impaired 15 . Similarly, cataract reversal individuals had a significantly reduced likelihood of showing the McGurk effect 16 . In this illusion, auditory speech, presented concurrently with an incongruent visual lip movement, produces a percept that matches neither the auditory nor the visual input. The absence of the McGurk effect in congenital cataract reversal individuals was subsequently shown to be related to a lack of multisensory enhancement in superior temporal brain regions, known to be essential for audio-visual speech perception 17 , 18 , 19 . Finally, visual motion after-effects (perception of stationary stimuli as moving in the direction opposite to previously presented moving stimuli) were found after auditory motion adaptation in cataract-reversal individuals 20 , i.e., a cross-modal after-effect which has not been found in normally sighted individuals. These results converge to the conclusion that multisensory binding processes do not fully recover after sight restoration in individuals with a history of congenital cataracts. Although recent studies with cataract reversal individuals have demonstrated recovery of multisensory redundancy effects 2 , 21 and partial recovery for auditory-visual simultaneity judgements 22 , multisensory binding based on more complex features, such as speech 14 , seems to depend on early visual input.

It is currently unclear to what degree visuo-haptic and visuo-motor processing recovers after a transient phase of congenital visual deprivation. A developmental study with children found that visuo-haptic integration reaches adult-like performance only by 10 years of age 23 . Prior to that, children show signs of either vision or touch dominating visuo-tactile perception. Evidence from studies in a small number of individuals who had dense (but not necessarily congenital) bilateral cataracts suggested a quick emergence of visuo-haptic interactions after surgery, when these individuals were tested in vision-to-touch object matching tasks (n = 1 24 ; n = 5 25 ). However, later single case studies pointed towards impaired spatial representations for visuo-tactile localization after at least two years of visual deprivation 26 , 27 . Further, a study testing sight recovery individuals on an automatic imitation task, which mapped vision to motoric performance, found performance deficits even two years after surgery 28 .

A phenomenon observed in typical visuo-haptic development is the perception of the size-weight illusion (henceforth referred to as the SWI), which has been described as “immutable” 29 . The SWI is an illusion perceived when two unequally sized objects of the same weight are compared—the smaller object is perceived as being heavier than the larger one.

The “classical” SWI is assumed to require the integration of visual and haptic input (for review, see Dijker) 30 . Though the SWI has been documented for a long time, there is still a debate on whether it occurs due to conflicting sensorimotor input, or is a purely cognitive effect due to a mismatch in expectations 31 . At present, the contribution of early visual experience to the occurrence of the illusion is unclear.

Several lines of evidence have suggested a crucial role of ongoing visual input in the perception of the SWI. First, the SWI was reported to disappear when visual cues were not presented to normally sighted participants, even if they were allowed to access them beforehand, suggesting a crucial role of continued visual perception for the illusion, and providing a strong argument that the SWI reflects visuo-haptic integration 32 . Additionally, the SWI was observed to increase with an increase in visual disparity between sizes; if two objects of the same weight had a greater difference between their visually perceived sizes, the illusion perceived was stronger 33 . This was found to be true even in the absence of haptically perceived size differences, when visually perceived size was varied using objects with adjustable heights but constant surface area 34 . Finally, in a rapid adaptation study using functional MRI with an SWI task, the ventral premotor area (PMv) responded more when the SWI was perceived, i.e. when participants compared the weights of two objects of different sizes and the same weight, than when participants compared objects of the same size and weight 35 . Importantly, the PMv did not show an independent adaptation to size and weight properties, but adapted to the combination of these properties, therefore providing neural evidence for the integration of concurrent but separate sensory input. These results suggest that early visual deprivation might affect the SWI, if the ability to integrate visual and haptic cues does not recover.

The SWI was observed to occur when size information was perceived exclusively visually (i.e. using a string set-up to weigh objects, preventing individuals from using haptic cues to estimate size) or exclusively haptically (i.e. blindfolding individuals to prevent them from accessing visual estimates of size) 36 , 37 . However, both these studies reported that the SWI was smaller when the task presented sighted individuals with size cues that were exclusively visual, as compared to when the task was haptic or visuo-haptic. Moreover, congenitally blind individuals have been reported to experience the SWI as well 37 , 38 . These results would lead us to hypothesize that the SWI emerges independent of visual input, and thus, should manifest unimpaired in sight-recovery individuals too—at least if the objects are perceived exclusively haptically. However, there might be some competition between belatedly available visual input and other sensory modalities 39 , 40 , which might worsen visual performance 14 , 20 . Consistent with this assumption, a reduced visuo-haptic SWI was reported in visually impaired individuals with some residual visual capabilities 41 . Therefore, it could be hypothesized that the visuo-haptic SWI, both with and without haptic size information, is reduced in individuals who recover from transient congenital or developmental visual deprivation.

The second account for the occurrence of the SWI has proposed that this illusion is a result of violated expectations, and originates from top-down rather than bottom up processes 42 , 43 , 44 , 45 . If the SWI is a result of exclusively visual statistics gathered over the lifespan—i.e. an increased amount of force on the larger object because of the belief that smaller objects should weigh less—then individuals who have not had access to vision early in life, when tested with and without haptic size information, should either not manifest the illusion, or perceive it to a lower degree.

In order to test for the dependence of visuo-haptic interactions on early visual input, we performed two experiments using an SWI paradigm employed by Buckingham and Goodale, wherein subjects were asked to rate cubes across three sizes (Small, Medium, Large) and two weights (350 g, 700 g) on how heavy they thought they were 42 . The first experiment tested the “classic” visuo-haptic SWI, that is the SWI when simultaneously receiving visual and haptic size information. A group of dense bilateral congenital cataract reversal individuals (CC) as well as a group of individuals who had suffered developmental cataracts (DC) were tested, and compared to a group of normally sighted controls (SC). We additionally ran and separately analyzed data from two congenitally blind (CB) individuals to pilot whether we would replicate the findings from Ellis and Lederman 37 . Such an illusion would necessarily be based on exclusively haptic estimates of size. The second experiment used a string set-up to ensure only visual size cues were available to participants, in order to isolate the visual contribution to the SWI 36 , 37 , 46 . A group of congenital cataract reversal individuals (CC) and a group of normally sighted controls (SC) were compared. We hypothesized that in Experiment 1, the SWI would be impaired in CC compared to SC and DC individuals, due to aberrant visual experience interfering with multisensory integration 39 , 40 , and that in Experiment 2, CC individuals would not experience the SWI when deprived of haptic size information 37 , 46 .

Sight-recovery individuals show an intact visuo-haptic SWI (Experiment 1)

Experiment 1 allowed participants to access both visual and haptic estimates of size (Fig.  1 A). When z-scored weight ratings were assessed in a size-by-weight-by-group analysis of variance (ANOVA with repeated measures), all groups performed the task in a principled manner, as indicated by a main effect of weight, wherein the 700 g weight was rated as heavier than the 350 g weight (F(1,23) = 639.15, p < 0.001, η g 2  = 0.85).

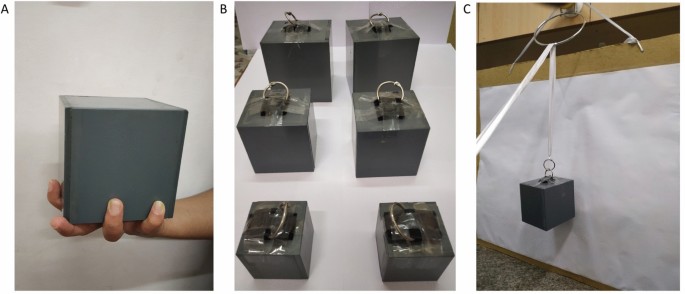
( A ) Large-sized cube stimulus placed in the dominant hand, demonstrating the procedure for Experiment 1. ( B ) The six stimuli used in the study (front to back: Small, Medium, Large, 350 g and 700 g respectively), with attachments for Experiment 2. ( C ) String set-up of Experiment 2. A smooth ribbon is used to lift the cube during each trial, ensuring that only visual size cues are available to the participant.

There was a main effect of size, demonstrating the presence of the SWI (F(2,46) = 147.11, p < 0.001, η g 2  = 0.74), i.e. participants perceived smaller sized objects of the same weight to be heavier. Crucially, the group-by-size ( F (4,46) = 0.96, p  = 0.419, η g 2  = 0.03), group-by-weight ( F (2,23) = 2.63, p  = 0.094, η g 2  = 0.04), and size-by-weight-by-group interactions ( F (4,46) = 1.64, p  = 0.181, η g 2  = 0.05) were all not significant. Therefore, CC individuals displayed an indistinguishable SWI from DC and SC individuals (Fig.  2 ).

Experiment 1 (with visual and haptic size information); z-scored weight ratings of all individuals in the Sighted Control (SC, yellow), the Developmental Cataract group (DC, blue), the Congenital Cataract group (CC, orange), and two Congenitally Blind (CB, red) individuals, t ( A ) for the 350 g and ( B ) for 700 g weights (Experiment 1).

We found a size-by-weight interaction, indicating that the illusion was stronger for the 700 g than the 350 g weight ( F (2,46) = 11.82, p  = 0.001, η g 2  = 0.15). In order to follow up on this effect, two post-hoc 3 (size) × 3 (group) repeated measures ANOVAs were conducted, separated by the weight condition. There was a main effect of size for both the 350 g ( F (2,46) = 59.08, p  < 0.001, η g 2  = 0.66) and the 700 g ( F( 2,46) = 108.24, p  < 0.001, η g 2  = 0.79) weights, demonstrating that the SWI was highly significant for both weights in all groups. Again, we found no group-by-size interaction (350 g: F (4,46) = 0.57, p  = 0.63, η g 2  = 0.04; 700 g: F (4,46) = 1.74, p  = 0.179, η g 2  = 0.11), indicating that the degree to which the three groups experienced the SWI was indistinguishable (Fig.  3 ).

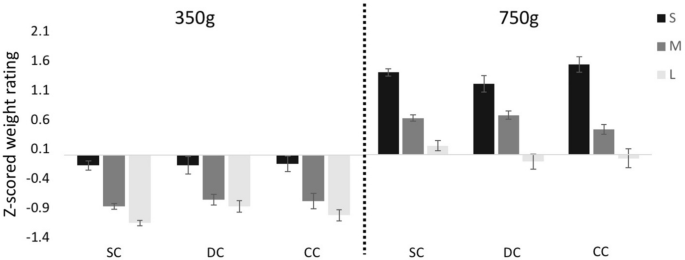
Experiment 1 (with visual and haptic size information); average z-scored weight ratings for the Small (S), Medium (M) and Large (L) cubes across the Sighted Control (SC), Developmental Cataract (DC) and Congenital Cataract (CC) groups, for the 350 g and 700 g weights (Experiment 1). Error bars depict standard error of mean (SEM).

Paired t-tests across the three groups confirmed that participants experienced the smaller cube as heavier than the medium (350 g: t (24) = 8.28, p  < 0.001; 700 g: t (24) = 7.64, p  < 0.001) and large cube (350 g: t (24) = 9.95, p  < 0.001; 700 g: t (24) = 11.52, p  < 0.001) of the same weight, and the medium sized cube as heavier than the large cube of the same weight (350 g: t (24) = 5.97, p  < 0.001; 700 g: t (24) = 8.49, p  < 0.001).

Both CB individuals tested showed an impressively clear SWI (Fig.  2 ). We found that their z-scores (Fig.  2 ) fell within the core range of the remaining groups. Thus, these two participants replicated prior results 37 , 46 .

In order to obtain a measure of illusion strength for each individual, we calculated an SWI Index by subtracting the mean z-scored weight judgment of the largest cube from that of the smallest cube, separately for each weight.

We conducted separate one way ANOVAs for the 350 g and 700 g weights to assess the effect of group on the calculated SWI Index. The CC, DC, and SC groups did not differ in the strength of the illusion—neither for the 350 g ( F (2,23) = 0.72, p  = 0.497, η g 2  = 0.06) nor the 700 g weights ( F (2,23) = 0.74, p  = 0.487, η g 2  = 0.06) (Fig.  3 ). We further tested these null findings against effect sizes obtained from studies in the literature. Based on a comparable study and task found for the 700 g weight, we used equivalence analyses 47 to confirm that the SWI index was equivalent in the CC, DC and SC groups—i.e. the SWI indices were within the bounds obtained by Buckingham and Goodale 42 (the presence of any meaningful effect of group was rejected with all p’s < 0.024, see Supporting Information S1 ).

The two CB individuals tested showed SWI indices within the range of the other groups (350 g: − 1.145 and − 1.034; 700 g: − 1.507 and − 0.646), demonstrating a full strength SWI.

Sight recovery individuals show an SWI with exclusively visual size estimates (Experiment 2)

Experiment 2 was performed as a follow up to the results from Experiment 1, in order to assess the occurrence of the SWI in the absence of haptic size cues (Fig.  1 B,C). Since we found no group differences in Experiment 1, and our a priori hypothesis was restricted to the effects of transient congenital patterned visual deprivation on the development of the SWI, we ran two groups: CC and SC individuals. Further, given the limitations on recruitment of special populations, as our goal with this experiment was to isolate the visual contribution to the full-sized SWI observed in CC individuals, DC individuals were not tested. In light of the occurrence of a full-sized SWI with (necessarily) exclusively haptic size cues in CB individuals in Experiment 1 and prior studies 37 , 46 , we did not repeat an additional haptics-only condition.

In a group-by-weight-by-size ANOVA performed for Experiment 2, we obtained a main effect of weight (F(1,11) = 110.80, p < 0.001, η g 2  = 0.833), indicating that participants performed the task in a principled manner. Additionally, the main effect of size was significant (F(2,22) = 8.82, p = 0.009, η g 2  = 0.203), confirming an SWI with this task across groups (Fig.  4 ). The CC participants did not differ from the SC group in their performance on this task, as evidenced by the lack of a significant main effect of group (F(1,11) = 0.158, p = 0.999, η g 2  < 0.001), and of significant group-level interactions (group-by-size: F(2,22) = 0.048, p = 0.861, η g 2  = 0.002; group-by-weight: F(1,11) = 1.866, p = 0.199, η g 2  = 0.077; group-by-size-by-weight: F(2,22) = 0.556, p = 0.509, η g 2  = 0.009).

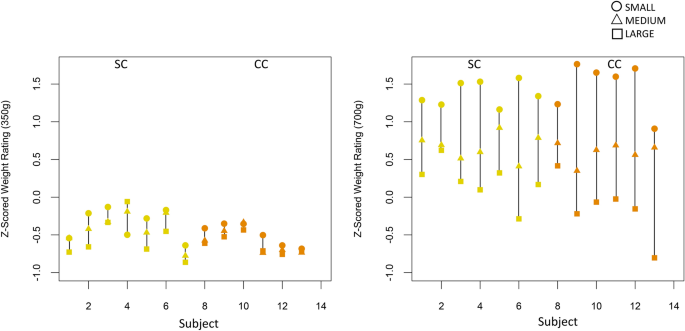
Experiment 2 (with only visual size information); z-scored weight ratings of all individuals in the Sighted Control group (SC, yellow) and Congenital Cataract group (CC, orange) groups (A) for the 350 g and (B) for 700 g weights (Experiment 2).

Across groups, participants experienced the smaller cube as heavier than the medium cube (350 g: t(12) = 2.03, p = 0.056; 700 g: t(12) = 2.02, p = 0.066) as well as the large cube (350 g: t(12) = 2.74, p = 0.018; 700 g: t(12) = 2.86, p = 0.014) of the same weight, and the medium sized cube as heavier than the large cube of the same weight (350 g: t(12) = 2.62, p = 0.023; 700 g: t(12) = 3.03, p = 0.010), confirming the presence of the SWI with this paradigm (Fig.  5 ).

Experiment 2 (with only visual size information); average z-scored weight ratings for the Small (S), Medium (M) and Large (L) cubes for the Sighted Control group (SC) and Congenital Cataract group (CC), for the 350 g and 700 g weights. Error bars depict standard error of mean (SEM).

The CC and SC groups did not differ in the strength of the illusion for either the 350 g ( F (1,11) = 0.169, p  = 0.688, η g 2  = 0.015), or the 700 g weights ( F (1,11) = 0.001, p  = 0.971, η g 2  < 0.001) (Fig.  5 ). When compared using equivalence testing for the 700 g weight, conducted based on available effect sizes in the literature, we found the SWI indices to be equivalent, significantly rejecting any effect of group (both p ’s < 0.006, see Supporting Information S1 ).

The visual contribution to the SWI is identical in sight recovery and sighted individuals

We compared the SC and CC groups of Experiment 1 and 2 in a group-by-weight-by-experimental task ANOVA, in order to assess whether the groups differed between how they performed when both visual and haptic size estimates were available, compared to when only visual size information was available. We confirmed that the SWI was significantly stronger in Experiment 1 compared to Experiment 2 (main effect of Experimental Task: F (1,26) = 56.396, p  < 0.001, η g 2  = 0.511), and stronger for the 700 g than the 350 g weight (main effect of Weight: F (1,26) = 9.089, p  = 0.006, η g 2  = 0.153) (Fig.  6 ). The group-by-experiment interaction ( F (1,26) = 0.357, p  = 0.555, η g 2  = 0.006), group-by-weight interaction ( F (1,26) = 1.083, p  = 0.307, η g 2  = 0.021), and group-by-weight-by-experiment interaction were all non-significant ( F (1,26) = 0.631, p  = 0.424, η g 2  = 0.012), confirming that in both experiments CC individuals perceived an indistinguishably strong SWI from SC individuals, suggesting that the “visual” contribution to the SWI did not differ in the two groups.

Size-Weight Illusion Indices across groups and for the two CB individuals for Experiment 1 (with visual and haptic size information, black bars, labelled VH) and Experiment 2 (with only visual size information, gray bars, labelled V), for the 350 g and 700 g weights. The y-Axis depicts the difference in z-scores between the large and small cubes of each weight, averaged for each group (CC, DC, and SC). Error bars depict the SEM.

Relationship between strength of the SWI and the duration of visual deprivation

To test for a possible effect of duration of patterned visual deprivation on the strength of the SWI, we calculated the correlation between age at surgery and the average SWI Index (across 350 g and 700 g) for CC individuals. This correlation was not significant neither for Experiment 1 (r = 0.05, t (4) = 0.10, p  = 0.463), nor did the correlation reach significance for Experiment 2 (r = − 0.76, t (4) = − 2.357, p  = 0.078). Additionally, no correlation was observed between illusion size and age at time tested in either group (see Supporting Information S4 .3).

The present study investigated whether the manifestation of the size-weight illusion (SWI) depends on patterned visual experience after birth. We tested sight recovery individuals with a history of dense bilateral congenital cataracts, and compared this group to sight recovery individuals with a history of developmental cataracts, as well as a group of normally sighted controls. Our results demonstrated a significant “classical” SWI (with visual and haptic size information available) in all groups; indeed, the size of the SWI was indistinguishable between the three groups. Furthermore, we replicated previous results from Ellis and Lederman 37 and showed that two permanently congenitally blind individuals experienced the SWI to a degree that fell within the range of all the other participants.

We additionally used a string set-up in Experiment 2 to test whether the CC group used visual size cues, rather than relying only on haptic size cues in Experiment 1 46 . As in Experiment 1, the SWI experienced with exclusively visual size information was equivalent across sight recovery individuals with a history of congenital cataracts, and sighted controls. Together, these results suggest that the visuo-haptic SWI is resilient to atypical visual experience after birth.

No sensitive period effects for visuo-haptic integration as tested by the SWI

A previous study of individuals who were operated upon for dense bilateral congenital cataracts reported that in an object matching task conducted two days-post-surgery, while unimodal tactile and visual performance was observed to be at ceiling, tactile to visual mapping was found to be severely impaired (Held et al., n = 5) 48 . However, the authors observed that this ability rapidly improved over the next five days. A subsequent case study of sight recovery suggested that visuo-tactile processing recovers in object recognition and object matching tasks within three days of sight restoration, despite a lack of visual experience after birth (Chen et al., n = 1) 24 . As these studies tested participants closer to the date of surgery, and given that the sight recovery individuals in the present study were all tested one year from surgery (in order to exclude acute but transient effects of surgery) our findings are consistent with these existing studies on visuo-haptic object recognition. However, both Held et al. and Chen et al. tested visuo-haptic transfer through object matching tasks. By contrast, we provide evidence for the recovery of visuo-haptic integration (i.e. a unified percept by fusing input from both sensory modalities) despite early patterned visual deprivation, therefore extending these studies 40 , 49 . Our findings might be considered surprising in light of two prospective studies, which suggested a protracted developmental pathway for the SWI. The first study observed that the SWI increased in size after the age of 5 years 43 . They related this increase to the development of abstract reasoning skills. However, abstract reasoning explained no more than about 10% of the effect, and the SWI existed even in the youngest group. A second study showed that typically developing children did not optimally integrate visuo-haptic input in an adult-like manner until the age of 10 years 23 . However, optimal integration is typically defined as optimal cue integration as predicted by forced fusion models. These models weight individual cues according to their relative reliability, to derive a multisensory outcome. It has more recently been demonstrated that in situations where it is ambiguous whether or not to integrate sensory information, the data from children as young as 5 years of age, like those of adults, are better explained by causal inference models 50 . Given that some (n = 4) of our CC participants had been older than 10 years of age at the time of surgery, our results suggest that patterned vision during this period of multisensory development was not crucial for the typical manifestation of the SWI, with either visuo-haptic or only visual size information.

These results showing an indistinguishable SWI in sight recovery individuals, both with a congenital as well as a developmental history of transient blindness, provide evidence that the multisensory processes underlying the SWI are resilient to atypical visual experience. First, participants of both cataract groups still suffered visual impairments at the time of testing. Nevertheless, the SWI was not smaller in magnitude in either group, compared to normally sighted individuals. A smaller SWI in sight recovery individuals would have been expected from the aforementioned reliability-based optimal integration account 51 . Second, neither years of blindness nor the timing of the transient phase of blindness (developmental vs. congenital) had a significant influence on the size of the SWI. Finally, the extent of the “visual” contribution to the SWI was indistinguishable between sight recovery and sighted individuals. This identical behavioral performance, regardless of atypical visual history across groups and tasks, provides strong evidence that visuo-haptic processing, as assessed with the SWI, does not rely on sensitive period plasticity to develop normally.

It is possible that different underlying neural mechanisms support the identically sized SWI in sight recovery individuals 52 . As sensitive periods are properties of neural circuits, further neuroimaging studies need to confirm whether visuo-haptic processing develops normally in the absence of typical visual experience 6 , 53 . Additionally, the absence of sensitive period effects in one tested behavior does not contradict the general role of sensitive period plasticity 5 , 53 , 54 , 55 , 56 . Disengaging functions which do and do not develop within sensitive periods will, in the long run, be essential to uncovering the general principles of functional brain development.

To the best of our knowledge, the present study is the first to conclusively show the manifestation of a full-strength SWI in sight recovery individuals, both for the condition with visual and haptic size information, as well as the condition with only visual size information. Further, strict criteria were used for the inclusion of sight recovery participants, in order to ensure high homogeneity of etiology within the CC and DC group. We chose a retrospective, developmental approach with individuals who underwent cataract reversal surgery before the age of 23 years, and were older than 8 years of age at the time of testing. After the age of 8 years, no further increase in the SWI had been observed in prospective studies 43 . The present study did not find any difference in the size of the SWI, neither for the visuo-haptic nor for the visual experiment. A significant, equivalent SWI was consistently perceived by individual participants across all groups, despite the fact that in the cataract groups, age at surgery, time since surgery and visual acuity at time of testing varied, potentially increasing between group differences. Our stringent inclusion criteria restricted the sample size within a special population, however, all individual subjects showed the SWI with established paradigms in a consistent pattern (Figs.  2 , 3 ), allowing us to interpret the lack of group differences and confirming them with equivalence testing. We consider these results to be highly robust evidence against the dependence of the SWI on early visual input, therefore suggesting the lack of a sensitive period for the development of the SWI.

Mechanisms of the SWI

What are the possible mechanisms by which the SWI could manifest in CC individuals? While our study does not allow us to disentangle the models explaining the occurrence of the SWI, interpreting our results in light of these hypotheses can shed light on the mechanisms of the SWI. Below, we engage with the dominant models of the SWI.

On one hand, it could be assumed that the SWI is a purely haptic process, as was consistent with earlier reports of the SWI manifesting in congenitally blind adults, and replicated in two congenitally bind adults in the present study 37 , 38 . However, a purely haptic account of the SWI would have predicted the absence of the SWI when haptic size cues are unavailable to sight recovery individuals. In fact, prior studies employing a string set-up in congenitally blind individuals, as expected, did not observe an SWI 37 , 46 . Instead, in the present study, sight recovery individuals perceived an SWI even when only visual size cues were available. Additionally, a recent study observed that congenitally blind individuals experience the SWI without haptic size cues, but when size estimates were obtained through echolocation 46 . Together, this evidence strongly argues against an exclusively haptic account of the SWI.

On the other hand, it has been suggested that the SWI is a multisensory phenomenon occurring due to a conflict between concurrent visual (size) and haptic (weight) sensory input 30 , 33 , 57 . Within this framework, our data suggest that while the SWI can develop through haptic input alone, it might nevertheless be modulated by visual input, due to the recovery of visuo-haptic processing despite atypical visual experience 22 , 24 , 25 . Indeed, full or partial recovery of visuo-tactile functions  has been reported, depending on the task. While prior studies have shown that in a simultaneity judgement task designed to test a unified multisensory percept, visuo-tactile performance was unimpaired despite a lack of early visual experience 21 , 22 , sight recovery individuals did not show normal visuo-tactile temporal order biases 27 , 58 . Additionally, our findings of a larger SWI when both visual and haptic size information was available than when only a visual size estimate was possible, in both sight recovery and sighted individuals, fit with a multisensory framework for the occurrence of the SWI 36 , 37 .

Conclusions

The occurrence of the Size-Weight Illusion (SWI), both when visual and haptic size information was available, as well as when only visual size information was assessable, was resilient to atypical visual experience within the first months and years of life. These results provide strong evidence that the visuo-haptic processes underlying the SWI do not require typical visual experience within a sensitive period for normal development. Further studies are needed to explore whether the SWI is supported by the same neural mechanisms in typical and atypical development, by employing neuroscience techniques 35 .

Ethical approval

All participants, as well as their legal guardians in case of minors, provided written and informed consent. Testing was conducted after obtaining ethical approval from the German Psychological Society (DGP) and the local ethics board of the Hyderabad Eye Research Foundation. All methods and tests were performed in accordance with the relevant guidelines and regulations of both collaborating institutions.

Experiment 1

Participants.

We tested three groups of individuals. The first group consisted of seven individuals born with dense bilateral cataracts, who subsequently underwent cataract removal surgery (referred to as CC individuals: 3 females, 4 males; Age = 8–35 years, M = 21.2 years, SD = 9.8, Table 1 ). The CC individuals were diagnosed by ophthalmologists and optometrists at the LV Prasad Eye Institute (LVPEI) in Hyderabad (India). They were tested by the some of the authors, partially with the help of a translator, in English, Hindi or Telugu. The data from four additional CC individuals were excluded due to unwillingness to cooperate (n = 2) or a documented developmental delay (n = 2). Individuals were categorized as part of this group based on the presence of dense bilateral cataracts at birth, a pre-surgery visual acuity of counting fingers at 1 m or less (barring absorption of lenses), presence of nystagmus, occlusion of fundus/retina, and immediate family members who had also been diagnosed with dense bilateral congenital cataracts. Duration of blindness was calculated by subtracting the date of birth from the date of the first eye surgery (M = 13.21 years, SD = 8.24, Range = 2–23.05 years). One individual did not have his precise date of surgery information available (operated after 6 months of age), and was excluded from duration calculations. Visual acuity pre-surgery in the better eye ranged from a minimum of light perception (PL +) to a maximum of counting fingers close to the face (CFCF). One participant had been able to count fingers at a distance of 3 m pre-surgery. We included this participant due to clearly partially absorbed lenses (OD Visual Acuity: counting fingers at 1.5 m, OS Visual Acuity: counting fingers at 3 m). All other criteria such as nystagmus and family history pointed towards the presence of dense bilateral cataracts at birth. Visual acuity post-surgery in the better eye in this group ranged from a minimum of counting fingers at a distance of 1 m to a maximum of 20/40. All individuals included in this group lacked patterned vision at birth, in accordance with the criteria set by the WHO 59 .

The second group consisted of nine individuals who had either partial congenital cataracts or developmental cataracts, and were subsequently operated upon to remove the cataracts (referred to as DC individuals: 2 females, 7 males; Age = 8–37 years, M = 14.8 years, SD = 9.2, Table 1 ). The testing procedure was the same as that of the CC group. Comparing this group with the CC individuals allowed us to isolate effects caused by transient patterned visual deprivation  at birth from effects due to general visual impairments caused by a changed periphery. Visual acuity pre-surgery in the better eye ranged from following light to a maximum of 20/80. Visual acuity post-surgery in the better eye ranged from 20/1200 to 20/20. All individuals included in this group did not lack patterned vision at birth, but suffered from degraded visual input for some or all of their early childhood, therefore providing a control group for the possibility that any observed impairments of the CC group were not specific to visual input at birth, but due to degraded visual input at any stage of life.

The third group consisted of 10 individuals with normal or corrected-to-normal vision and who had no history of visual deficits or eye injuries (referred to as SC group: 7 females 3 males; Age = 19–36 years, M = 25.8 years, SD = 5.3). SC individuals were tested at the University of Hamburg, Hamburg, Germany, in German.

In addition to these three groups, we ran two congenitally blind individuals who had no more than light perception since birth and at the time of the study (referred to as CB individuals: 1 female, 1 male; Ages = 33 and 44 years). They were tested at the University of Hamburg, Germany, using German. Their data were analyzed separately due to the small group size. The purpose of including these two participants was to replicate the presence of the SWI in individuals who totally lack vision since birth, but not for statistical comparisons between groups 37 , 46 .

All individuals included in the data analysis reported no history of neurological or cognitive impairments. All participants were right handed, and used that hand to perform the task.

Stimuli and apparatus

Participants were tested using a free-rating, absolute-magnitude-estimation procedure: they freely chose a rating scale of their preference and estimated how much an object weighed on that scale 42 . This scale was adjustable during the course of the experiment.

Participants rated one of 6 gray plastic cubes that were placed on their palms. The cubes were small (5 cm side length), medium (7.5 cm side length) or large (10 cm side length), and had one of two different weights (either 350 g or 700 g) (Fig.  1 ). The weight was invisibly fixed in one corner, with the rest of the inside being hollow to ensure the same distribution of weight in each cube. Participants were instructed to hold the dominant arm bent at 90 degrees, and for each trial, the cube was placed on the palm of their dominant hand such that it was clearly visible. Participants were required to lift each cube for approximately 15 s, and to judge its weight. Upon the rating response, the experimenter removed the cube from the participants’ hand.

We used random orders, and in one run, each cube was lifted 5 times. We repeated this across two runs, leading to a total of 60 trials. For participants who did not complete 60 trials, we used only the completed run of 30 trials (CC: n = 1; DC: n = 3). Participants were instructed not to rotate the arm for additional sensory cues, and not to throw the cube in the air and catch it again. This procedure took around 30 minutes in total.

Data analysis

In order to compare subjective judgements across participants, rating scores were z-transformed within each participant. This was done by subtracting the individual’s mean weight judgement from each weight judgement, and dividing by the standard deviation 42 . Due to this z-scoring, all weight judgements reflect deviations from the same mean (zero), with higher z values indicating heavier weight judgements. In order to be included in the data analysis, participants had to consistently rate the 350 g cubes as lighter than the 700 g cubes, to exclude the possibility of a response bias as opposed to a principled difference in perceived weight due to size. This was true of all participants in Experiment 1.

We used frequentist statistics to analyze the data. Z-scores across participants were submitted to a mixed ANOVA. Our model considered two within-group factors—namely, weight (2 levels: 350 g, 700 g) and size (3 levels: small, medium, large), and one between-group factor—group (3 levels: CC, DC, and SC) in a repeated measures ANOVA. Levene’s test for Homogeneity of Variance was performed on the z-score data to ensure that the scores do not violate the assumption of equal variance for parametric testing, due to unequal sample sizes between groups ( F( 2,23) = 0.27, p  = 0.764). Post-hoc ANOVAs and t-tests were performed according to the resulting interactions, and post-hoc equivalence testing was conducted to confirm the results (Supplementary Information S1 ).

All analyses were conducted in R (version 3.3.2), using the ez-package ( https://github.com/mike-lawrence/ez ). This package corrects for violations of sphericity when there are more 2 levels in the within subject variable (size) via the Greenhouse–Geisser correction. All effect sizes reported are generalized eta squared (η g 2 ) values.

This study was not pre-registered, and sample sizes were limited by strict inclusion criteria within a special population.

Experiment 2

Data for this experiment was collected at LVPEI, Hyderabad, India, by the some of the authors, partially with the help of a translator, in English, Hindi or Telugu.

The CC group consisted of six individuals defined and classified the same way as in Experiment 1 ( 1 female, 5 males; Age = 17–44.7 years, M = 27.67 years, SD = 12.37; Duration of blindness = 2–22.01 years, M = 12.98 years, SD = 7.28, Table 1 ). An additional CC participant was excluded as they did not consistently rate the 350 g cubes as less heavy than the 700 g cubes, indicating that they were not performing the task in a principled manner, possibly due to translation issues (see Supporting Information S2 ). For four out of six included participants, visual acuity pre-surgery in the better eye ranged from a minimum of counting fingers at 1 m to a maximum of 20/300, with a history of partially absorbed lenses in all four of them. All other criteria, such as presence of nystagmus and family history, pointed towards the presence of dense bilateral cataracts at birth. For the remaining two participants, visual acuity pre-surgery was unknown, but based on the combination of a family history of dense congenital cataracts, very poor visual acuity post-surgery, nystagmus and esotropia, these participants were classified as having dense bilateral congenital cataracts. Visual acuity post-surgery in the better eye in this group ranged from a minimum of 20/400 to a maximum of 20/125.

The SC group consisted of seven individuals with normal or corrected-to-normal vision, with no history of eye injuries or abnormalities (5 females, 2 males; Age = 21–29 years, M = 24.13, SD = 3.08).

Participants used a white, smooth ribbon to hold and lift one of the same six cubes used in Experiment 1, by pulling it with their dominant hand. The ribbon was wrapped around a metal ring fixed to a wall, in order to minimize friction that could possibly affect weight judgements, and allow participants to estimate the weight of the cube while it was suspended at eye level (Fig.  1 ). An important experimental consideration for the use of a string/handle set-up to test the SWI in sight recovery individuals was to control for the precision of the visual cues provided, due to residual visual impairments in visually impaired individuals 5 . Therefore, viewing distance was determined by each CC and SC participant based on how comfortable they were seeing the cube. However, viewing distance was not significantly different between groups (t(4) = 1.656, p = 0.137; CC: Mean = 58 cm, SD = 8.69 cm, Range = 50–70 cm; SC: Mean = 64.71 cm, SD = 5.19 cm, Range = 60–73 cm). This was done to minimize the possibility of potential differences in illusion size being confounded with differences in visual acuity, due to viewing at a fixed distance. Participants were instructed identically to Experiment 1 described above, and the experimenter placed and removed the cubes from the apparatus for each trial to prevent the participant from having any haptic contact with the stimuli.

Participants were not permitted to haptically handle the cubes at any time, before or during the course of the task, and were naïve to how many sizes and weights were presented. A post-study questionnaire recorded their estimates for how many weights and sizes were presented (see Supporting Information S3 ).

The same random trial orders used in Experiment 1 were used for Experiment 2, and 60 trials were completed per participant.

Z-scores were calculated using a procedure identical to the one described for Experiment 1 above.

The ANOVA model comprised two within-group factors (Size: 3 levels, Weight: 2 levels) and one between-group factor with 2 levels (CC, SC). Post-hoc ANOVAs and t-tests were performed according to the resulting interactions.

Additionally, a cross-experiment ANOVA with group and experimental task as between- subject factors and weight as a within- subject factor (group: 2 levels for SC and CC; task: 2 levels for Experiment 1 and 2) was performed.

Data availability

The datasets generated/analyzed during this study are available from the corresponding author upon reasonable request.

Birch, E. E., Stager, D., Leffler, J. & Weakley, D. Early treatment of congenital unilateral cataract minimizes unequal competition. Investig. Ophthalmol. Vis. Sci. 39 , 1560–1566 (1998).

CAS   Google Scholar  

de Heering, A. et al. A brief period of postnatal visual deprivation alters the balance between auditory and visual attention. Curr. Biol. https://doi.org/10.1016/j.cub.2016.10.014 (2016).

Article   PubMed   Google Scholar  

Maurer, D. Critical periods re-examined: Evidence from children treated for dense cataracts. Cogn. Dev. https://doi.org/10.1016/j.cogdev.2017.02.006 (2017).

Article   Google Scholar  

Lewkowicz, D. J. & Röder, B. The effects of experience on the development of multisensory processing. New Handb. multisensory Process. (2015).

Lewis, T. L. & Maurer, D. Multiple sensitive periods in human visual development: Evidence from visually deprived children. Dev. Psychobiol. 46 , 163–183 (2005).

Knudsen, E. I. Sensitive periods in the development of the brain and behavior. J. Cogn. Neurosci. 16 , 1412–1425 (2004).

Maurer, D. & Lewis, T. L. Visual Systems. Neurobiol. Brain Behav. Dev. https://doi.org/10.1016/B978-0-12-804036-2.00008-X (2017).

Eagleman, D. M. Visual illusions and neurobiology. Nat. Rev. Neurosci. https://doi.org/10.1038/35104092 (2001).

Putzar, L., Hötting, K., Rösler, F. & Röder, B. The development of visual feature binding processes after visual deprivation in early infancy. Vis. Res. https://doi.org/10.1016/j.visres.2007.07.002 (2007).

McKyton, A., Ben-Zion, I., Doron, R. & Zohary, E. The limits of shape recognition following late emergence from blindness. Curr. Biol. https://doi.org/10.1016/j.cub.2015.06.040 (2015).

Hadad, B. S., Maurer, D. & Lewis, T. L. The development of contour interpolation: Evidence from subjective contours. J. Exp. Child Psychol. https://doi.org/10.1016/j.jecp.2010.02.003 (2010).

Hadad, B. S., Maurer, D. & Lewis, T. L. The role of early visual input in the development of contour interpolation: the case of subjective contours. Dev. Sci. https://doi.org/10.1111/desc.12379 (2017).

Gandhi, T., Kalia, A., Ganesh, S. & Sinha, P. Immediate susceptibility to visual illusions after sight onset. Curr. Biol. https://doi.org/10.1016/j.cub.2015.03.005 (2015).

Article   PubMed   PubMed Central   Google Scholar  

Putzar, L., Goerendt, I., Lange, K., Rösler, F. & Röder, B. Early visual deprivation impairs multisensory interactions in humans. Nat. Neurosci. https://doi.org/10.1038/nn1978 (2007).

Meredith, M. & Stein, B. Interactions among converging sensory inputs in the superior colliculus. Science https://doi.org/10.1126/science.6867718 (1983).

Putzar, L., Hötting, K. & Röder, B. Early visual deprivation affects the development of face recognition and of audio-visual speech perception. Restor. Neurol. Neurosci. https://doi.org/10.3233/RNN-2010-0526 (2010).

Nath, A. R. & Beauchamp, M. S. A neural basis for interindividual differences in the McGurk effect, a multisensory speech illusion. Neuroimage https://doi.org/10.1016/j.neuroimage.2011.07.024 (2012).

Kumar, G. V. et al. Large scale functional brain networks underlying temporal integration of audio-visual speech perception: An eeg study. Front. Psychol. https://doi.org/10.3389/fpsyg.2016.01558 (2016).

Sams, M. et al. Seeing speech: Visual information in the human auditory cortex. Neurosci. Lett. 127 , 141–145 (1991).

Article   CAS   Google Scholar  

Guerreiro, M. J. S., Putzar, L. & Röder, B. Persisting cross-modal changes in sight-recovery individuals modulate visual perception. Curr. Biol. https://doi.org/10.1016/j.cub.2016.08.069 (2016).

Putzar, L., Gondan, M. & Rder, B. Basic multisensory functions can be acquired after congenital visual pattern deprivation in humans. Dev. Neuropsychol. https://doi.org/10.1080/87565641.2012.696756 (2012).

Chen, Y. C., Lewis, T. L., Shore, D. I. & Maurer, D. Early binocular input is critical for development of audiovisual but not visuotactile simultaneity perception. Curr. Biol. https://doi.org/10.1016/j.cub.2017.01.009 (2017).

Gori, M., Del Viva, M., Sandini, G. & Burr, D. C. Young children do not integrate visual and haptic form information. Curr. Biol. https://doi.org/10.1016/j.cub.2008.04.036 (2008).

Chen, J. et al. Rapid integration of tactile and visual information by a newly sighted child. Curr. Biol. https://doi.org/10.1016/j.cub.2016.02.065 (2016).

Held, R. et al. The newly sighted fail to match seen with felt. Nat. Neurosci. https://doi.org/10.1038/nn.2795 (2011).

Azañón, E., Camacho, K., Morales, M. & Longo, M. R. The sensitive period for tactile remapping does not include early infancy. Child Dev. https://doi.org/10.1111/cdev.12813 (2018).

Ley, P., Bottari, D., Shenoy, B. H., Kekunnaya, R. & Röder, B. Partial recovery of visual-spatial remapping of touch after restoring vision in a congenitally blind man. Neuropsychologia https://doi.org/10.1016/j.neuropsychologia.2013.03.004 (2013).

McKyton, A., Ben-Zion, I. & Zohary, E. Lack of automatic imitation in newly sighted individuals. Psychol. Sci. https://doi.org/10.1177/0956797617731755 (2018).

Murray, D. J., Ellis, R. R., Bandomir, C. A. & Ross, H. E. Charpentier (1891) on the size-weight illusion. Percept. Psychophys. https://doi.org/10.3758/BF03213127 (1999).

Dijker, A. J. M. The role of expectancies in the size-weight illusion: A review of theoretical and empirical arguments and a new explanation. Psychon. Bull. Rev. https://doi.org/10.3758/s13423-014-0634-1 (2014).

Flanagan, J. R., Bittner, J. P. & Johansson, R. S. Experience can change distinct size-weight priors engaged in lifting objects and judging their weights. Curr. Biol. https://doi.org/10.1016/j.cub.2008.09.042 (2008).

Masin, S. C. & Crestoni, L. Experimental demonstration of the sensory basis of the size-weight illusion. Percept. Psychophys. https://doi.org/10.3758/BF03210411 (1988).

Kawai, S., Henigman, F., MacKenzie, C. L., Kuang, A. B. & Faust, P. H. A reexamination of the size-weight illusion induced by visual size cues. Exp. Brain Res. https://doi.org/10.1007/s00221-006-0803-1 (2007).

Plaisier, M. A. & Smeets, J. B. J. Object size can influence perceived weight independent of visual estimates of the volume of material. Sci. Rep. https://doi.org/10.1038/srep17719 (2015).

Chouinard, P. A., Large, M. E., Chang, E. C. & Goodale, M. A. Dissociable neural mechanisms for determining the perceived heaviness of objects and the predicted weight of objects during lifting: An fMRI investigation of the size-weight illusion. Neuroimage https://doi.org/10.1016/j.neuroimage.2008.08.023 (2009).

Pick, H. L. & Pick, A. D. A developmental and analytic study of the size-weight illusion. J. Exp. Child Psychol. https://doi.org/10.1016/0022-0965(67)90064-1 (1967).

Article   PubMed   MATH   Google Scholar  

Ellis, R. R. & Lederman, S. J. The role of haptic versus visual volume cues in the size-weight illusion. Percept. Psychophys. https://doi.org/10.3758/BF03205186 (1993).

Rice, J. The size–weight illusion among the blind. Amm. Psychol. Assn. 27 , 81–87 (1898).

Google Scholar  

Guerreiro, M. J. S., Putzar, L. & Röder, B. The effect of early visual deprivation on the neural bases of multisensory processing. Brain https://doi.org/10.1093/brain/awv076 (2015).

Singh, A. K., Phillips, F., Merabet, L. B. & Sinha, P. Why does the cortex reorganize after sensory loss?. Trends Cogn. Sci. https://doi.org/10.1016/j.tics.2018.04.004 (2018).

Furth, H. G. Effect of training on the adaptation level of the size-weight illusion with normal, deaf, and blind subjects. Percept. Mot. Skills 13 , 155–160 (1961).

Buckingham, G. & Goodale, M. A. Lifting without seeing: The role of vision in perceiving and acting upon the size weight Illusion. PLoS ONE https://doi.org/10.1371/journal.pone.0009709 (2010).

Chouinard, P. A. et al. The development of the size–weight illusion in children coincides with the development of nonverbal cognition rather than motor skills. J. Exp. Child Psychol. https://doi.org/10.1016/j.jecp.2019.03.006 (2019).

Peters, M. A. K., Balzer, J. & Shams, L. Smaller = denser, and the brain knows it: Natural statistics of object density shape weight expectations. PLoS ONE https://doi.org/10.1371/journal.pone.0119794 (2015).

Ross, H. E. Sensory information necessary for the size-weight illusion [58]. Nature https://doi.org/10.1038/212650a0 (1966).

Buckingham, G., Milne, J. L., Byrne, C. M. & Goodale, M. A. The size-weight illusion induced through human echolocation. Psychol. Sci. https://doi.org/10.1177/0956797614561267 (2015).

Lakens, D. Equivalence tests: A practical primer for t tests, correlations, and meta-analyses. Soc. Psychol. Personal. Sci. https://doi.org/10.1177/1948550617697177 (2017).

Held, R. et al. The newly sighted fail to match seen with felt. Nat. Neurosci. 14 , 551–553 (2011).

Stein, B. E. et al. Semantic confusion regarding the development of multisensory integration: A practical solution. Eur. J. Neurosci. https://doi.org/10.1111/j.1460-9568.2010.07206.x (2010).

Rohlf, S., Li, L., Bruns, P. & Röder, B. Multisensory integration develops prior to crossmodal recalibration. Curr. Biol. https://doi.org/10.1016/j.cub.2020.02.048 (2020).

Ernst, M. O. & Banks, M. S. Humans integrate visual and haptic information in a statistically optimal fashion. Nature https://doi.org/10.1038/415429a (2002).

Bedny, M. Evidence from blindness for a cognitively pluripotent cortex. Trends Cogn. Sci. https://doi.org/10.1016/j.tics.2017.06.003 (2017).

Takesian, A. E. & Hensch, T. K. Balancing plasticity/stability across brain development. Prog. Brain Res. https://doi.org/10.1016/B978-0-444-63327-9.00001-1 (2013).

Röder, B., Ley, P., Shenoy, B. H., Kekunnaya, R. & Bottari, D. Sensitive periods for the functional specialization of the neural system for human face processing. Proc. Natl. Acad. Sci. U. S. A. https://doi.org/10.1073/pnas.1309963110 (2013).

Hadad, B. S., Maurer, D. & Lewis, T. L. Sparing of sensitivity to biological motion but not of global motion after early visual deprivation. Dev. Sci. https://doi.org/10.1111/j.1467-7687.2012.01145.x (2012).

Röder, B., Kekunnaya, R. & Guerreiro, M. J. S. Neural mechanisms of visual sensitive periods in humans. Neurosci. Biobehav. Rev. https://doi.org/10.1016/j.neubiorev.2020.10.030 (2020).

Grandy, M. S. & Westwood, D. A. Opposite perceptual and sensorimotor responses to a size-weight illusion. J. Neurophysiol. https://doi.org/10.1152/jn.00851.2005 (2006).

Badde, S. et al. Sensory experience during early sensitive periods shapes cross-modal temporal biases. Elife https://doi.org/10.7554/ELIFE.61238 (2020).

World Health Organisation. World Report on Vision (World Health Organisation, Geneva, 2019).

Download references

Acknowledgements

We are grateful to Dr. D. Balasubramanian and the LVPEI for supporting the study at the LVPEI in Hyderabad. We thank Dr. Kabilan Pitchaimuthu, Sven Leach and Dr. Suddha Sourav for helping with the data acquisition. Dirk Waschatz provided technical assistance. The study was funded by the German Research Foundation (DFG Ro 2625/10-1) and the European Research Council grant ERC-2009-AdG 249425-CriticalBrainChanges to Dr. Brigitte Röder. Rashi Pant was supported by a PhD student fellowship from the Hector Fellow Academy gGmbH.

Open Access funding enabled and organized by Projekt DEAL.

Author information

Authors and affiliations.

Biological Psychology and Neuropsychology, University of Hamburg, 20146, Hamburg, Germany

Rashi Pant, Maria J. S. Guerreiro, Pia Ley, Davide Bottari & Brigitte Röder

Child Sight Institute, Jasti V Ramanamma Children’s Eye Care Center, LV Prasad Eye Institute, Hyderabad, Telangana, 500034, India

Idris Shareef & Ramesh Kekunnaya

Molecular Mind Lab, IMT School for Advanced Studies, 55100, Lucca, Italy

Davide Bottari

You can also search for this author in PubMed   Google Scholar

Contributions

R.P. designed and collected data for Experiment 2, analyzed the data for both experiments, made the figures and tables and wrote the paper. M.G., P.L. and D.B. designed and collected data for Experiment 1, M.G. analyzed the data for both experiments and wrote the paper. I.S. recruited, counselled and diagnosed sight recovery individuals and assisted in data collection for Experiment 2. R.K. counselled and diagnosed sight recovery individuals for all experiments and supervised the work. B.R. designed the study, collected the data for Experiment 1 and wrote the paper. All authors edited the manuscript.

Corresponding author

Correspondence to Rashi Pant .

Ethics declarations

Competing interests.

The authors declare no competing interests.

Additional information

Publisher's note.

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Information

Supplementary information., rights and permissions.

Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/ .

Reprints and permissions

About this article

Cite this article.

Pant, R., Guerreiro, M.J.S., Ley, P. et al. The size-weight illusion is unimpaired in individuals with a history of congenital visual deprivation. Sci Rep 11 , 6693 (2021). https://doi.org/10.1038/s41598-021-86227-w

Download citation

Received : 28 March 2019

Accepted : 10 March 2021

Published : 23 March 2021

DOI : https://doi.org/10.1038/s41598-021-86227-w

Share this article

Anyone you share the following link with will be able to read this content:

Sorry, a shareable link is not currently available for this article.

Provided by the Springer Nature SharedIt content-sharing initiative

This article is cited by

• Sophia Piller
• Irene Senna
• Marc O. Ernst

Scientific Reports (2023)

Quick links

• Explore articles by subject
• Guide to authors
• Editorial policies

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.